
Consider the charges q, q and –q placed at the vertices of the equilateral triangle, as shown in the figure. What is the force on each charge?

Answer
443.4k+ views
Hint: Emissive power is the radiant energy emitted by the Body. Body should be anything either it is black or gray, coefficient of emission is the fractional part of power of a perfectly black Body at the same temperature.
Formula Used: -
Where,
K is the Coulomb’s constant
Complete step by step answer:
The electric force between two static and charged particles is called an electrostatic force. The nature of this force can be attractive or repulsive depending on whether the particles are positively/negatively charged. Coulomb’s law provides us the equation which relates the force and the charged particles along with the distance in the given charged particles.
In this question the length of the equilateral triangle is not given. Therefore, we will assume it to be l.
Now from Coulomb’s law
We know,
Now, let us calculate the force on first particle 1 say
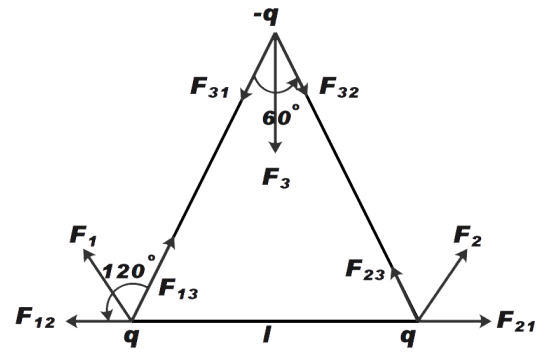
Now, we know that particles with the same charge repel each other and that with opposite charges attract each other. Therefore, as shown in figure
Now for, the charge on both particles is equal in magnitude as well as direction and distance is taken as l
Therefore, Coulomb’s law can be modified as
After substituting the values
We get,
Let,
Therefore,
Similarly, for force
After substituting values,
We get,
Similarly, if we calculate the magnitude of other forces say
We will get the same result
Therefore,
Now we know that
Therefore, from (2) and (3)
We get,
On solving
We get,
Now, since the distance between the charge 1 and 2 is the same, also their charge and other factors such as angle and magnitude of component forces is the same. From the figure, we can say that
Therefore,
Now, for charge 3, we can say that angle between the component vector force say
Therefore, the magnitude resultant force
On solving
We get,
Now, from (1) we know that
Therefore, from (1), (A) and (B)
We get,
Also from (1) and (C
We get,
Therefore, force on charged particle q, q and –q is
Note:
The Coulomb’s constant k is given as,
Where,
Formula Used: -
Where,
K is the Coulomb’s constant
Complete step by step answer:
The electric force between two static and charged particles is called an electrostatic force. The nature of this force can be attractive or repulsive depending on whether the particles are positively/negatively charged. Coulomb’s law provides us the equation which relates the force and the charged particles along with the distance in the given charged particles.
In this question the length of the equilateral triangle is not given. Therefore, we will assume it to be l.
Now from Coulomb’s law
We know,
Now, let us calculate the force on first particle 1 say

Now, we know that particles with the same charge repel each other and that with opposite charges attract each other. Therefore, as shown in figure
Now for, the charge on both particles is equal in magnitude as well as direction and distance is taken as l
Therefore, Coulomb’s law can be modified as
After substituting the values
We get,
Let,
Therefore,
Similarly, for force
After substituting values,
We get,
Similarly, if we calculate the magnitude of other forces say
We will get the same result
Therefore,
Now we know that
Therefore, from (2) and (3)
We get,
On solving
We get,
Now, since the distance between the charge 1 and 2 is the same, also their charge and other factors such as angle and magnitude of component forces is the same. From the figure, we can say that
Therefore,
Now, for charge 3, we can say that angle between the component vector force say
Therefore, the magnitude resultant force
On solving
We get,
Now, from (1) we know that
Therefore, from (1), (A) and (B)
We get,
Also from (1) and (C
We get,
Therefore, force on charged particle q, q and –q is
Note:
The Coulomb’s constant k is given as,
Where,
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
EMI starts from ₹3,775 per month
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




