Question

Calculate the charge on a capacitor in a steady-state.

a) 50$\mu C$
b) 30$\mu C$
c) 45$\mu C$
d) 60$\mu C$

Answer 1

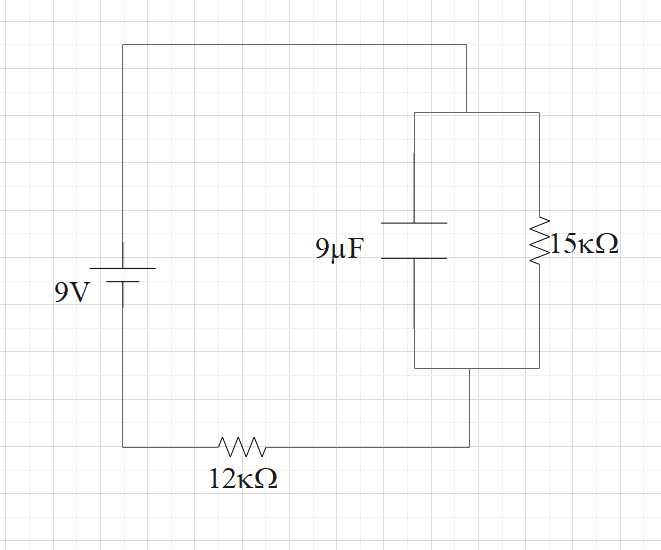
Hint: In a steady-state, the capacitor becomes open. So, considering the diagram given, find the current in the circuit where the resistors are connected in series, using Ohm's law: $V=IR$, where V is voltage, I is current through circuit and R is total resistance. Now, we know that the capacitor and resistor are connected parallelly. So, the voltage across them remains the same. So, using the current found in the circuit and the resistance is given, calculate the voltage across the capacitor.
As we know that the voltage across a capacitor is given by $V=\dfrac{q}{C}$, where q is the charge on the capacitor and C is the capacitance. Use the formula to find the charge on the capacitor.

Complete step by step answer:
From the diagram shown above, we know that the capacitor becomes zero in steady-state and the resistors are connected in series.
So, we need to calculate the total resistance by using the series formula: $R={{R}_{1}}+{{R}_{2}}$
So, we have:
$\begin{align}
  & R=\left( 15+12 \right)\times {{10}^{3}}\Omega \\
 & =27\times {{10}^{3}}\Omega
\end{align}$
Now, find the current in the circuit using Ohm’s Law: $V=IR$
We get:
$\begin{align}
  & I=\dfrac{V}{R} \\
 & =\dfrac{9}{27\times {{10}^{3}}}A......(1)
\end{align}$
Now, we know that the voltage across the capacitor and the resistor ${{R}_{1}}$ is same, as they are connected in parallel. So, voltage across capacitor = voltage across resistor ${{R}_{1}}$
So, voltage across resistor by using Ohm’s law is:
$\begin{align}
  & V=I{{R}_{1}} \\
 & =\dfrac{9}{27\times {{10}^{3}}}\times 15\times {{10}^{3}} \\
 & =5V
\end{align}$
Therefore, voltage across the capacitor is 5V.
Now, we know that, the voltage across the capacitor is given as: $V=\dfrac{q}{C}$
For given values of V=5V and C = $9\mu F$, we get the value of capacitance as:
$\begin{align}
  & q=VC \\
 & =5\times 9\times {{10}^{-6}} \\
 & =45\times {{10}^{-6}}C
\end{align}$

So, the correct answer is “Option C”.

Note:
Be careful while applying series and parallel formulas for calculating the resistance. Students might make mistakes with the formula. As well as, do remember which quantity remains the same in both the configurations.