
By using integration prove that the area of a circle of radius r units is
Answer
528k+ views
Hint: Use the fact that the equation of a circle of radius r centred at the origin is
Complete step-by-step answer:
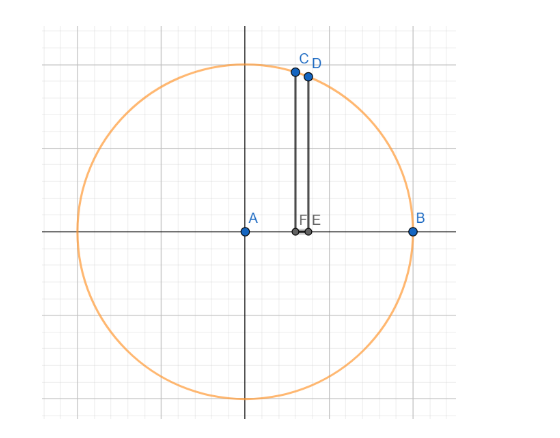
As is evident from the graph that the curve is symmetrical in the four quadrants. Hence, we will find the area in the first quadrant, and then the total area will be four times the area in the first quadrant.
Now, we have
Subtracting
Hence, we have
Since in the first quadrant
Now consider the vertical strip CDEF.
Here CF = y and EF = dx.
Hence the area of the strip is ydx.
The total area in the first quadrant will be the sum of these vertical strips from A to B.
At point B, we have
y =0
Hence, we have
Since the abscissa of B is positive, we have
Hence, we have
The area in the first quadrant is
Substituting the value of y, we get
The area in the first quadrant is
Let
Finding the value of I:
Let x = r sint
Differentiating both sides with respect to t, we get
When x = 0, we have
When x = r, we have
Hence, we have
We know that
Hence, we have
Since in the interval
Hence, we have
We know that
Hence, we have
We know that
Hence, we have
Adding equation (i) and equation (ii), we get
We know that
Hence, we have
Dividing both sides by 2, we get
Hence the area in the first quadrant is
Hence the area of the circle is
Q.E.D
Note:[1] We can directly solve I by using the fact that
Hence, we have
Which is the same as obtained above.
Complete step-by-step answer:

As is evident from the graph that the curve is symmetrical in the four quadrants. Hence, we will find the area in the first quadrant, and then the total area will be four times the area in the first quadrant.
Now, we have
Subtracting
Hence, we have
Since in the first quadrant
Now consider the vertical strip CDEF.
Here CF = y and EF = dx.
Hence the area of the strip is ydx.
The total area in the first quadrant will be the sum of these vertical strips from A to B.
At point B, we have
y =0
Hence, we have
Since the abscissa of B is positive, we have
Hence, we have
The area in the first quadrant is
Substituting the value of y, we get
The area in the first quadrant is
Let
Finding the value of I:
Let x = r sint
Differentiating both sides with respect to t, we get
When x = 0, we have
When x = r, we have
Hence, we have
We know that
Hence, we have
Since in the interval
Hence, we have
We know that
Hence, we have
We know that
Hence, we have
Adding equation (i) and equation (ii), we get
We know that
Hence, we have
Dividing both sides by 2, we get
Hence the area in the first quadrant is
Hence the area of the circle is
Q.E.D
Note:[1] We can directly solve I by using the fact that
Hence, we have
Which is the same as obtained above.
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 12 Biology: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




