
Average voltage for the given source is
A)
B)
C)
D)

Answer
503.7k+ views
Hint: The average voltage of a periodic waveform like this triangular one can be defined as the quotient of the area under the waveform with respect to time. To calculate you need to average out all the instantaneous values along the time axis with time being one full period which for such a continuous waveform is calculated by calculating the area under the curve.
Formula used:
If a triangle has a base
Complete step by step answer:
Given:
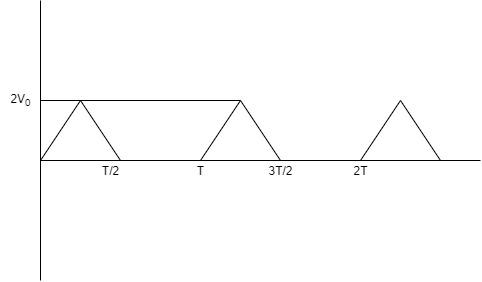
The peak of the voltage from the triangular waveforms shown in the graph is
The period of the waveform from the graph is
To get: The average voltage
Step 1:
You can calculate the area under each periodic triangle from eq (1).
You can see for each triangle the base is
So, the area under each triangle is
Step 2:
So, the quotient of the area under the waveform with respect to time period
Calculate the average voltage
where
The average voltage for the given source is
Note:
Here you can approach to calculate the area under the curve through any method, but for the continuous waveform, you need to use this approach for the appropriate solution. One needs to be very careful in choosing the period. The symmetry of the wave form should be noticed for this type of regular waveform which can be easily computed.
Formula used:
If a triangle has a base
Complete step by step answer:
Given:
The peak of the voltage from the triangular waveforms shown in the graph is
The period of the waveform from the graph is
To get: The average voltage
Step 1:
You can calculate the area under each periodic triangle from eq (1).
You can see for each triangle the base is
So, the area under each triangle is
Step 2:
So, the quotient of the area under the waveform with respect to time period
Calculate the average voltage
where
The average voltage for the given source is
Note:
Here you can approach to calculate the area under the curve through any method, but for the continuous waveform, you need to use this approach for the appropriate solution. One needs to be very careful in choosing the period. The symmetry of the wave form should be noticed for this type of regular waveform which can be easily computed.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?




