
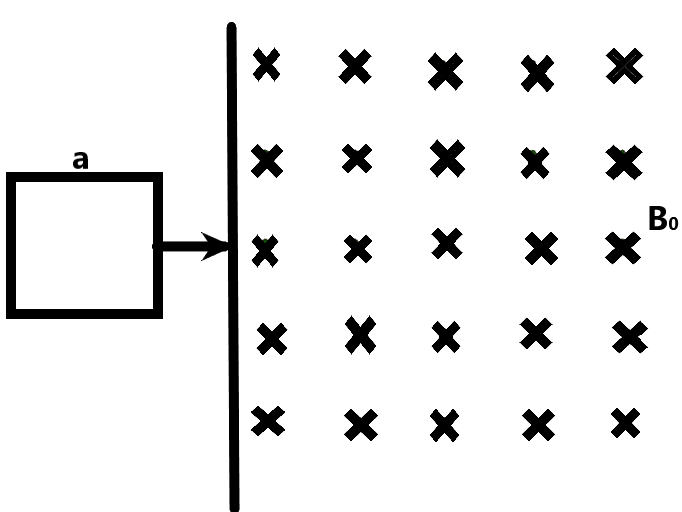
A square loop of side a, resistance R, mass m is sliding as shown on a smooth horizontal table with speed

Answer
492.6k+ views
Hint: We need to understand the factors that are affecting the motion of a resistor in a perpendicular magnetic field. We can relate the given quantities such as the magnetic field, resistance, and area of the loop to get the velocity of the square initially.
Complete step-by-step solution
We know that the magnetic field provides a force on a conducting material, which moves in the field with a perpendicular component of velocity to the direction of the magnetic field. We are given a square, which moves with its plane perpendicular to the direction of the magnetic field with an initial velocity.
The Lorentz force due to this motion experienced by the square loop is given by –
Where B is the magnetic field strength,
‘I’ is the induced current in the square loop.
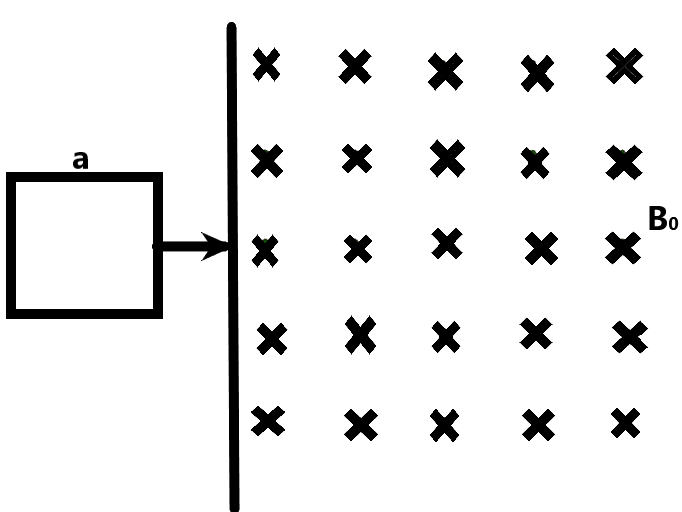
We know that the current induced in a loop is given by the relation –
Substituting this in the equation for force gives –
We can equate the force to the product of the mass and acceleration on the square loop as –
This is the required initial velocity for the square to travel a distance of ‘l’ in the magnetic field.
The correct answer is option A.
Note: The moving coil or the square loop should have a perpendicular component along the direction of the magnetic field for any Lorentz force act upon the conducting coil or the resistor. We needn’t require the angle here because all the velocity was along the perpendicular.
Complete step-by-step solution
We know that the magnetic field provides a force on a conducting material, which moves in the field with a perpendicular component of velocity to the direction of the magnetic field. We are given a square, which moves with its plane perpendicular to the direction of the magnetic field with an initial velocity.
The Lorentz force due to this motion experienced by the square loop is given by –
Where B is the magnetic field strength,
‘I’ is the induced current in the square loop.

We know that the current induced in a loop is given by the relation –
Substituting this in the equation for force gives –
We can equate the force to the product of the mass and acceleration on the square loop as –
This is the required initial velocity for the square to travel a distance of ‘l’ in the magnetic field.
The correct answer is option A.
Note: The moving coil or the square loop should have a perpendicular component along the direction of the magnetic field for any Lorentz force act upon the conducting coil or the resistor. We needn’t require the angle here because all the velocity was along the perpendicular.
Recently Updated Pages
Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




