
A charge q is placed at the centre of the line joining two exactly equal positive charges Q. The system of these charges will be in equilibrium, if q is equal to
Answer
450.7k+ views
8 likes
Hint: Use the coulomb’s law that gives the force between two charges. First, check what must be the nature of the third charge so that the three charges can be in equilibrium. Then find the net force on each charge and equate it to zero.
Formula used:
Complete answer:
Let two positive points sized charges of equal magnitude Q be placed in vacuum. Let the distance between the two charges be d. Now, we placed a third charge q at the midpoint of the line joining the two charges, i.e. at a distance of
Here, each charge will experience a force due to the other two charges. Now, for the system to be at equilibrium, the net forces on all the three charges must be zero.
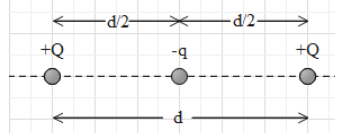
We know that two positive charges always repel and move away from each other. Therefore, the middle charge must be a negative charge so that it attracts the two charges and keeps them at rest.
Let them find the net force on each charge and equate it to zero. For this we will use the coulomb’s law that gives us the force between two charges of magnitudes
i.e.
Let us find the force on the middle negative charge due to the two positive charges. The force due to the both positive charges will be
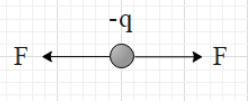
However, these two forces are in opposite directions. Hence, the net force on the middle charge will be zero.
Let us find the force on the positive charge at left due the negative charge and the other positive charge.
The positive charge will push away the charge this charge with a force
The negative charge will attract this charge with force
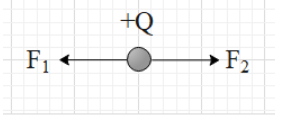
Hence, the net force on the charge is
For the positive charge to be at rest, the net force must be zero.
Similarly, for the other positive charge to be at rest, q must be
Therefore, the magnitude of the third charge must be
Therefore, the third is -
Hence, the correct option is A.
Note:
Note that like charges always repel each other and unlike charges attract each other.
This means that a positive charge will always attract a negative charge and vice versa.
However, when both the charges are either positive or negative, they repel each other.
Formula used:
Complete answer:
Let two positive points sized charges of equal magnitude Q be placed in vacuum. Let the distance between the two charges be d. Now, we placed a third charge q at the midpoint of the line joining the two charges, i.e. at a distance of
Here, each charge will experience a force due to the other two charges. Now, for the system to be at equilibrium, the net forces on all the three charges must be zero.
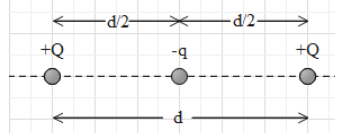
We know that two positive charges always repel and move away from each other. Therefore, the middle charge must be a negative charge so that it attracts the two charges and keeps them at rest.
Let them find the net force on each charge and equate it to zero. For this we will use the coulomb’s law that gives us the force between two charges of magnitudes
i.e.
Let us find the force on the middle negative charge due to the two positive charges. The force due to the both positive charges will be
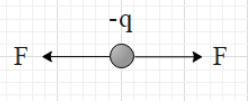
However, these two forces are in opposite directions. Hence, the net force on the middle charge will be zero.
Let us find the force on the positive charge at left due the negative charge and the other positive charge.
The positive charge will push away the charge this charge with a force
The negative charge will attract this charge with force

Hence, the net force on the charge is
For the positive charge to be at rest, the net force must be zero.
Similarly, for the other positive charge to be at rest, q must be
Therefore, the magnitude of the third charge must be
Therefore, the third is -
Hence, the correct option is A.
Note:
Note that like charges always repel each other and unlike charges attract each other.
This means that a positive charge will always attract a negative charge and vice versa.
However, when both the charges are either positive or negative, they repel each other.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




