Question

A $11.7ft$ wide ditch with the approach roads at an angle of 15° with the horizontal. With what minimum speed should a motorbike be moving on the road so that it safely crosses the ditch? Assume that the length of the bike is \[5{\text{ }}ft\], and it leaves the road when the front part runs out of the approach road.

Answer 1

Hint: In order to answer the question we will assume the bike is a point object, and the ditch is \[2.5{\text{ }} + {\text{ }}2.5 = 0.5\] feet wider, or \[16.7\]feet deep, so it leaves the road as the front portion runs out the approach road. Then, using the definition of a projectile, we will calculate the minimum speed with a projection angle of 15 degrees and a length of 16.7 feet.

Complete step by step answer:
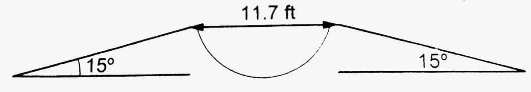
Given, Width of the ditch= $11.7ft$ and Length of the bike= $5ft$. The approach road makes an angle of ${15^ \circ }\left( \alpha \right)$ with the horizontal. The biker's total horizontal width that must be protected in order to safely cross the ditch,
$R = 11.7 + 5 = 16.7\,ft$
Acceleration due to gravity,
$a = g = 9.8\,m/s \\
= \,32.2\,ft/{s^2} \\ $
The horizontal range, as we know, is determined by
$R = \dfrac{{{u^2}\sin 2\alpha }}{g}$
We get, by placing respective values,
${u^2} = \dfrac{{Rg}}{{\sin 2\alpha }} \\
\Rightarrow {u^2}= \dfrac{{16.7 \times 32.2}}{{\sin {{30}^ \circ }}}$
$ \therefore u \approx 32ft/\operatorname{s} $

As a result, the minimum speed at which the motorcycle can travel is $32ft/s$.

Note:The trajectory equation is the equation of projectile motion. So, if we know the x-component of an object's location, we can use the projectile motion equation to find the y-component of the position. The equation of the direction of projectile motion can be used to obtain all of the quantities involved in projectile motion, either directly or indirectly.