
Write the statement of Gauss' law for electrostatics. Draw a diagram and derive an expression for the electric field due to a uniformly charged infinite plane sheet at a point near the sheet. For the given diagram, write the value of electric flux passing through the surface.


Answer
492.6k+ views
1 likes
Hint: The Gauss’s law relates the distribution of electric charge and the resulting electric field. To apply Gauss's law, we have to understand the quantity known as electric flux which is the integral of the electric field and area.
Complete step by step answer:
Part 1: Gauss’s law of electrostatics:
Gauss’ law gives a relationship between the electric flux through a closed surface and the amount of charge enclosed by it. For the expression of electric field due to a uniformly charged infinite plane sheet use gauss’ law around a cylindrical surface.
Gauss law for electrostatics states that total electric flux through a closed surface is the same as the enclosed charge by the surface divided by the permittivity of the medium. Mathematically:
Where,
Part 2
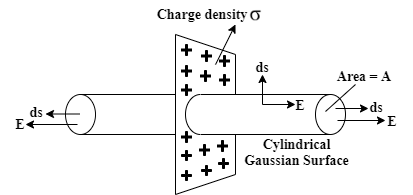
Let, Surface charge density of the sheet is
So, for an infinite charged sheet electric field is
Part 3

In the given diagram, total charge inside the closed surface is
Hence, the value of flux passing through the surface is approximately
Note: Although gauss law is valid for any kind of closed surface, but for practical application of gauss law you always need to be very careful about choosing the type of Gaussian surface. Otherwise, executing the surface integral becomes too hard. So, to make life easier choose the Gaussian surface wisely.
Complete step by step answer:
Part 1: Gauss’s law of electrostatics:
Gauss’ law gives a relationship between the electric flux through a closed surface and the amount of charge enclosed by it. For the expression of electric field due to a uniformly charged infinite plane sheet use gauss’ law around a cylindrical surface.
Gauss law for electrostatics states that total electric flux through a closed surface is the same as the enclosed charge by the surface divided by the permittivity of the medium. Mathematically:
Where,
Part 2

Let, Surface charge density of the sheet is
So, for an infinite charged sheet electric field is
Part 3

In the given diagram, total charge inside the closed surface is
Hence, the value of flux passing through the surface is approximately
Note: Although gauss law is valid for any kind of closed surface, but for practical application of gauss law you always need to be very careful about choosing the type of Gaussian surface. Otherwise, executing the surface integral becomes too hard. So, to make life easier choose the Gaussian surface wisely.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




