
What is the value of
Answer
432.6k+ views
Hint :First we know that a unit cell is the smallest portion of a crystal lattice that shows the three-dimensional pattern of the entire crystal. There are three types of unit cells: simple cubic (primitive cubic), Face-Centred Cubic (FCC) , and Body-Centred Cubic (BCC).
Complete Step By Step Answer:
A BCC unit cell has atoms at each corner of the cube and an atom at the centre of the structure. The diagram shown below is an open structure. According to this structure, the atom at the body centre wholly belongs to the unit cell in which it is present.
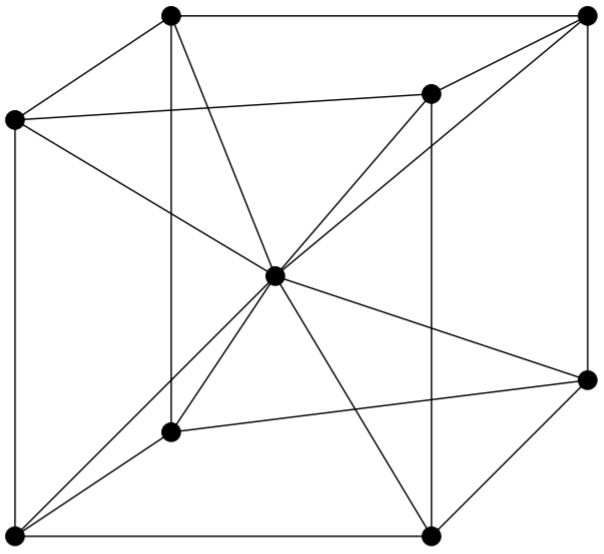
In the BCC unit cell every corner has atoms.
There is one atom present at the centre of the structure.
In the BCC cell we have,
Therefore, the total number of atoms present per unit cell
Since the number of atoms for a body-centred cubic unit cell is
Hence the value of
Note :
Alpha iron, Tungsten, Chromium, and Beta titanium are the examples of metals with the BCC structure. Note that a crystal can be thought of as the same unit cell repeated over and over in three dimensions. The number of atoms in a face-centred cubic unit cell is four. Also, the number of atoms in a simple cubic unit cell is one.
Complete Step By Step Answer:
A BCC unit cell has atoms at each corner of the cube and an atom at the centre of the structure. The diagram shown below is an open structure. According to this structure, the atom at the body centre wholly belongs to the unit cell in which it is present.

In the BCC unit cell every corner has atoms.
There is one atom present at the centre of the structure.
In the BCC cell we have,
Therefore, the total number of atoms present per unit cell
Since the number of atoms for a body-centred cubic unit cell is
Hence the value of
Note :
Alpha iron, Tungsten, Chromium, and Beta titanium are the examples of metals with the BCC structure. Note that a crystal can be thought of as the same unit cell repeated over and over in three dimensions. The number of atoms in a face-centred cubic unit cell is four. Also, the number of atoms in a simple cubic unit cell is one.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE

When was the first election held in India a 194748 class 12 sst CBSE




