
How do you use the Extreme Value Theorem to determine the global extrema of the function
Answer
446.1k+ views
Hint: First find the first derivative of the given function and equate it to zero to find the critical points. Consider the end points of the given interval. Find the value of the given function at each critical point and end points. The least of these values is the minimum and the greatest is the maximum.
Formula used:
Differentiation formula
Complete step by step solution:
The Extreme value theorem states that if a function is continuous on a closed interval
Given
Differentiating with respect to
Equating the equation (2) to zero to find the critical points, we get
Since
And consider the endpoints of the closed interval
Finding the values of the given function
Hence, from the above values. The maximum value and minimum value of the given function are
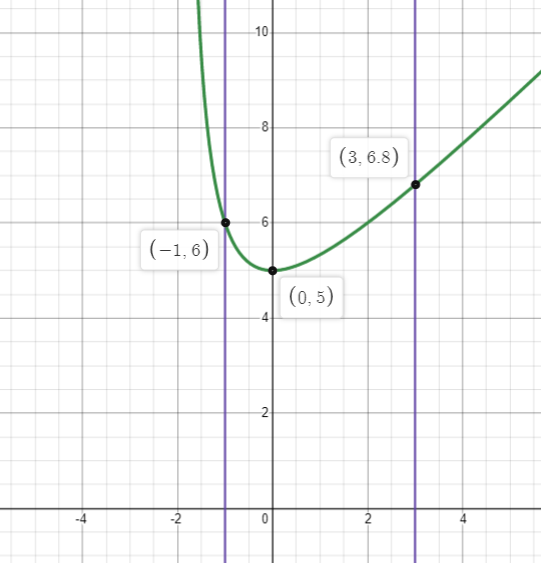
The graph of the function is:
(Note that the graph is in the whole range, observe the critical points in the range of [-1,3] as marked on the graph).
Note:
Note that a function is continuous if you can draw its graph without lifting the pencil. Maximum value and minimum value of the given function is also known as absolute maxima and absolute minima respectively.
Formula used:
Differentiation formula
Complete step by step solution:
The Extreme value theorem states that if a function is continuous on a closed interval
Given
Differentiating with respect to
Equating the equation (2) to zero to find the critical points, we get
Since
And consider the endpoints of the closed interval
Finding the values of the given function
Hence, from the above values. The maximum value and minimum value of the given function are
The graph of the function is:
(Note that the graph is in the whole range, observe the critical points in the range of [-1,3] as marked on the graph).

Note:
Note that a function is continuous if you can draw its graph without lifting the pencil. Maximum value and minimum value of the given function is also known as absolute maxima and absolute minima respectively.
Recently Updated Pages
Master Class 12 Biology: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Father of Indian ecology is a Prof R Misra b GS Puri class 12 biology CBSE

Who is considered as the Father of Ecology in India class 12 biology CBSE

Enzymes with heme as prosthetic group are a Catalase class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

An example of ex situ conservation is a Sacred grove class 12 biology CBSE

Why is insulin not administered orally to a diabetic class 12 biology CBSE




