
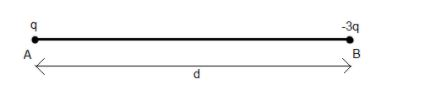
Two charges

Answer
513k+ views
2 likes
Hint: This problem can be solved by using Coulomb’s law for the force on the charge due to the other two charges. Unlike charges attract each other whereas like charges repel each other. Therefore, the positive charge
Formula used:
Complete step-by-step answer:
Since like charges attract repel each other while unlike charges attract each other, students must realize that if the third charge is placed in between the two charges, the repulsive force due to the positive charge and the attractive force due to the negative charge will be in the same direction and therefore, the charge will not be in equilibrium.
Therefore, we will place the charge at a distance
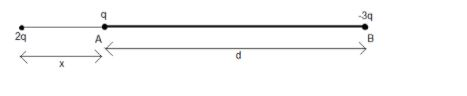
Now, the Coulomb force
Where
Now, using (1), we get the sum of the forces on the charge
Now, for equilibrium,
Square rooting both sides we get
Rationalizing, we get
Therefore, we have got the required expression for the distance from
Therefore, the correct option is
So, the correct answer is “Option B”.
Note: While putting the square root in the calculation, we neglected the negative root since doing that would mean that
Formula used:
Complete step-by-step answer:
Since like charges attract repel each other while unlike charges attract each other, students must realize that if the third charge is placed in between the two charges, the repulsive force due to the positive charge and the attractive force due to the negative charge will be in the same direction and therefore, the charge will not be in equilibrium.
Therefore, we will place the charge at a distance

Now, the Coulomb force
Where
Now, using (1), we get the sum of the forces on the charge
Now, for equilibrium,
Square rooting both sides we get
Rationalizing, we get
Therefore, we have got the required expression for the distance from
Therefore, the correct option is
So, the correct answer is “Option B”.
Note: While putting the square root in the calculation, we neglected the negative root since doing that would mean that
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




