
The voltage shown in the figure below is known as a triangular waveform. Determine its rms value.
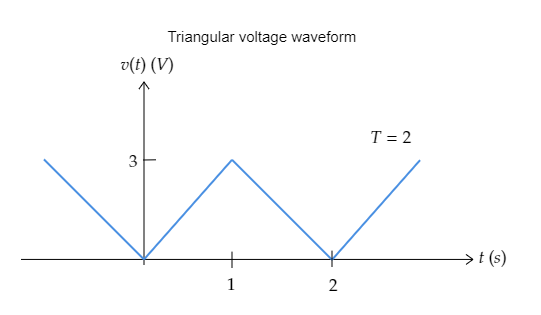

Answer
503.4k+ views
1 likes
Hint:The rms value refers to the effective value of a varying voltage or a varying current. Here we have to determine the rms voltage of the given triangular form. The rms value of any waveform refers to the root of the area under the squared waveform over the period of the waveform. For a sawtooth or triangular waveform, the rms value of the voltage is also given to be
Formulas used:
-The rms voltage of a triangular waveform is given by,
-The rms voltage of any waveform is given by,
-The area of a parabola is given by,
Complete step by step answer.
Step 1: Sketch the given triangular waveform and list its important parameters.
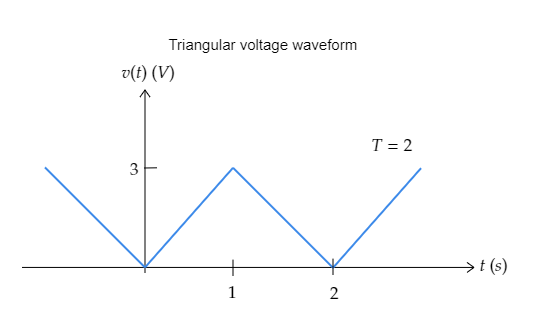
As seen from the above figure, the maximum voltage of the waveform is
The period of the waveform is mentioned to be
Step 2: Sketch the squared waveform of the given triangular wave and obtain its area.
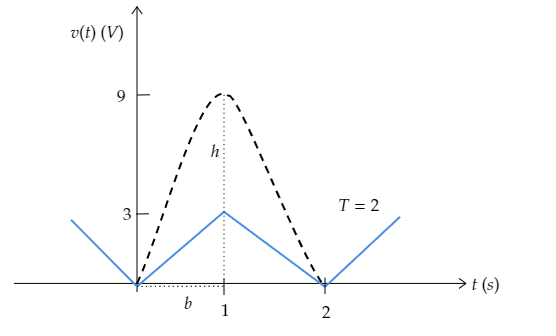
The vertical distance to the highest point in the square waveform will be the square of the maximum voltage of the triangular wave i.e.,
The horizontal distance to the highest vertical point on the waveform is observed to be
This squared waveform is a parabola.
The area of a parabola is given by,
From the figure, the area of the squared waveform will be
Substituting
Thus we have the area as
Step 3: Express the relation for the rms value of the voltage for a triangular voltage waveform.
Now the rms value of the triangular waveform is defined by,
Substituting for
Thus the rms value of the voltage is
Note: Alternate method
For a triangular voltage waveform, the rms value is given by,
Substituting in equation (A) we get,
Thus the rms value of the triangular voltage waveform is
Formulas used:
-The rms voltage of a triangular waveform is given by,
-The rms voltage of any waveform is given by,
-The area of a parabola is given by,
Complete step by step answer.
Step 1: Sketch the given triangular waveform and list its important parameters.

As seen from the above figure, the maximum voltage of the waveform is
The period of the waveform is mentioned to be
Step 2: Sketch the squared waveform of the given triangular wave and obtain its area.

The vertical distance to the highest point in the square waveform will be the square of the maximum voltage of the triangular wave i.e.,
The horizontal distance to the highest vertical point on the waveform is observed to be
This squared waveform is a parabola.
The area of a parabola is given by,
From the figure, the area of the squared waveform will be
Substituting
Thus we have the area as
Step 3: Express the relation for the rms value of the voltage for a triangular voltage waveform.
Now the rms value of the triangular waveform is defined by,
Substituting for
Thus the rms value of the voltage is
Note: Alternate method
For a triangular voltage waveform, the rms value is given by,
Substituting in equation (A) we get,
Thus the rms value of the triangular voltage waveform is
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE




