
The sum of the length, breadth and height of a cuboid is 38 cm and the lengths of its diagonal is 22 cm. Then find the total surface area of the cuboid.
Answer
536.4k+ views
Hint:- Formula for diagonal of the cuboid is
Complete step-by-step solution -
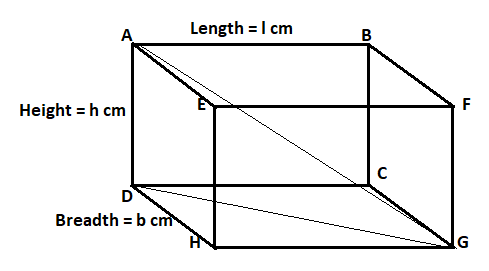
Now as we can see from the above figure that diagonal of the cuboid ABCDHGFE is the line joining the points A and D.
And as we know that a, b, c are the length, breadth and height of any cuboid then its diagonal length must be equal to
So, let the length of be AB = DC = EF = HG = l cm
Breadth of the cuboid will be AE = DH = BF = CG = b cm
And, the height of the cuboid will be equal to AD = EH = BC = FG = h cm.
And it is given that the sum of the length, breadth and height of the cuboid is 38 cm.
So, l + b + h = 38 cm (1)
And,
On squaring both sides of the above equation. We get,
Now as we know that the formula for total surface area is 2(ab + bc + ca). So, the total surface area of the above cuboid will be 2(lb + bh + hl).
So, to find the value of the total surface area of the cuboid we must use the identity of
So,
Now putting the value of
Now solving the above equation. We get,
Subtracting 484 to both sides of the above equation we get,
As RHS of the above equation is the formula for calculating the total surface area of a cuboid. So, LHS must be equal to the total surface area of the cuboid.
Hence, the total surface area of the cuboid is 960
Note:- The main diagonal of the cuboid is one which cuts the cuboid through the centre of it. And length if the diagonal is

Complete step-by-step solution -
Now as we can see from the above figure that diagonal of the cuboid ABCDHGFE is the line joining the points A and D.
And as we know that a, b, c are the length, breadth and height of any cuboid then its diagonal length must be equal to
So, let the length of be AB = DC = EF = HG = l cm
Breadth of the cuboid will be AE = DH = BF = CG = b cm
And, the height of the cuboid will be equal to AD = EH = BC = FG = h cm.
And it is given that the sum of the length, breadth and height of the cuboid is 38 cm.
So, l + b + h = 38 cm (1)
And,
On squaring both sides of the above equation. We get,
Now as we know that the formula for total surface area is 2(ab + bc + ca). So, the total surface area of the above cuboid will be 2(lb + bh + hl).
So, to find the value of the total surface area of the cuboid we must use the identity of
So,
Now putting the value of
Now solving the above equation. We get,
Subtracting 484 to both sides of the above equation we get,
As RHS of the above equation is the formula for calculating the total surface area of a cuboid. So, LHS must be equal to the total surface area of the cuboid.
Hence, the total surface area of the cuboid is 960
Note:- The main diagonal of the cuboid is one which cuts the cuboid through the centre of it. And length if the diagonal is
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
What is the Full Form of ISI and RAW

Which of the following districts of Rajasthan borders class 9 social science CBSE

Difference Between Plant Cell and Animal Cell

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

What is 85 of 500 class 9 maths CBSE




