
The radius of the largest sphere which fits properly at the center of the edge of body centered cubic unit cell is: (edge length is represented by a)
(A) 0.134a
(B) 0.027a
(C) 0.067a
(D) 0.047a
Answer
490.5k+ views
Hint: A unit cell is the smallest repeating unit in the crystal lattice, it is the building block of a crystal like the cell which is the building block of the human body. Body centered cubic cell is one of the types of the unit cell.
Complete step by step solution:
To solve this question we have to find the relation between the radius (r) and the edge of the body centered unit cell (a). We know that the total number of atoms present in the body centered cubic cell is = 2.
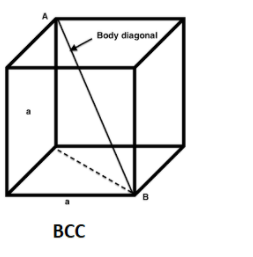
In body centered unit cells, atoms in the body diagonals are in contact with each other. So the length of the body diagonal is =
The body diagonal =
So, 4R =
According to the question
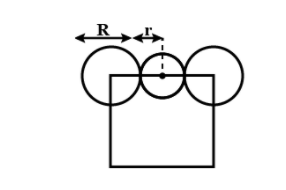
From equation 1 and 2
r = 0.067a
Hence the correct answer is option (C)
Note: In the body centered unit cell, atoms are present at the center and at the corner of the cube. And as we know that there is only one center in a cube hence only one atom is completely present there that means that this atom is not shared with any other unit cell and one atom at the corner contributes to eight other corners. So the total number of atoms present in the body centered cubic cell is = 2. i.e.
1 atom at body center
Complete step by step solution:
To solve this question we have to find the relation between the radius (r) and the edge of the body centered unit cell (a). We know that the total number of atoms present in the body centered cubic cell is = 2.

In body centered unit cells, atoms in the body diagonals are in contact with each other. So the length of the body diagonal is =
The body diagonal =
So, 4R =
According to the question

From equation 1 and 2
r = 0.067a
Hence the correct answer is option (C)
Note: In the body centered unit cell, atoms are present at the center and at the corner of the cube. And as we know that there is only one center in a cube hence only one atom is completely present there that means that this atom is not shared with any other unit cell and one atom at the corner contributes to eight other corners. So the total number of atoms present in the body centered cubic cell is = 2. i.e.
1 atom at body center
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE

When was the first election held in India a 194748 class 12 sst CBSE




