
The magnetic field at a distance x on the axis of a circular coil of radius R is
A)
B)
C)
D)
Answer
455.4k+ views
Hint: In the above question the magnetic field along the axis of the coil is at its centre is given to us. The magnetic field along the axis is governed by a particular relation. Hence comparing the magnetic field at the centre of the coil and at distance x such that the magnetic field is
Formula used:
Complete step-by-step answer:
Let us say we have a circular coil of radius R carrying current ‘i’ as shown in the figure below.
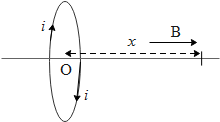
The magnetic field at a distance x from the centre of the coil is given by,
At x = 0, i.e. the centre of the coil the magnetic field is equal to,
Similarly the magnetic field at a distance x from the centre is equal to,
In the question it is given that the magnetic field at a distance x on the axis of a circular coil of radius R is
So, the correct answer is “Option C”.
Note: It is to be noted that we have taken the cube root on both the sides of the equation so that the power 3 on both the sides becomes 1. Further squaring on both the sides will help to get rid of the square root in the subsequent step. In the above question we have assumed the coil to be of single loop as the value of magnetic field in case of comparison does not matter.
Formula used:
Complete step-by-step answer:
Let us say we have a circular coil of radius R carrying current ‘i’ as shown in the figure below.

The magnetic field at a distance x from the centre of the coil is given by,
At x = 0, i.e. the centre of the coil the magnetic field is equal to,
Similarly the magnetic field at a distance x from the centre is equal to,
In the question it is given that the magnetic field at a distance x on the axis of a circular coil of radius R is
So, the correct answer is “Option C”.
Note: It is to be noted that we have taken the cube root on both the sides of the equation so that the power 3 on both the sides becomes 1. Further squaring on both the sides will help to get rid of the square root in the subsequent step. In the above question we have assumed the coil to be of single loop as the value of magnetic field in case of comparison does not matter.
Latest Vedantu courses for you
Grade 7 | ICSE | SCHOOL | English
Vedantu ICSE 7 Pro Course (2025-26)
School Full course for ICSE students
₹45,000 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE




