Question

The length of a rectangular park is 0.140km, and the breadth is 80m. What is the perimeter of the park? Three rounds of barbed wire are needed to fence the park. Find the cost of fencing the park at a rate of Rs 2.20 per metre.

Answer 1

Hint: Use the fact that the perimeter of a rectangle = 2(l+b) where l is the length of the rectangle and b is the breadth of the rectangle. Hence find the total length of barbed wire required to fence the rectangle three rounds with the barbed wire. Hence find the cost of fencing at the rate of Rs.2.20 per metre.

Complete step-by-step answer:

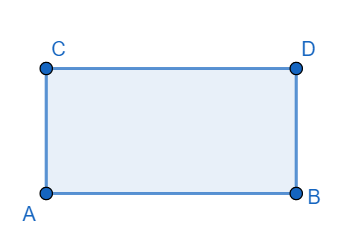
We have the length of the rectangle = l = AB = 0.140 km
Since 1km = 1000m, we have
AB = l = 140m
Also the breadth of the rectangle = AC = 80m.
Now, we have the perimeter of the rectangle = AB+AC+BD+CD
Since opposite sides of a parallelogram are equal, we have
AB = CD, AC = BD.
Hence the perimeter of the rectangle = l+b+l+b = 2l+2b.
Now l = 140 m and b = 80m
Hence the perimeter of the rectangle $=2\times 140+2\times 80=280+160=440m$
Hence the perimeter of the rectangle = 440m.
The length of the barbed wire to fence the rectangle three rounds $=440\times 3=1320m$
Hence the cost of fencing @Rs. 2.20/m $=1320\times 2.20=2904$
Hence the cost of fencing = Rs 2904

Note: In these types of questions take special care about the units of each of the given dimensions. It is very important to know the basic conversion of the units and making the units the same when calculating. Many students forget to do so and hence get incorrect results.