
The height of a cone is 10cm. The cone is divided into two parts by drawing a plane through the midpoint of the axis of the cone, parallel to the base. Compare the volume of the two parts.
A.1 : 7
B.2 : 9
C.3 : 11
D.3 : 5
Answer
545.4k+ views
Hint: volume of a cone with radius r and height h is equal to
Let the height of the given cone be h cm.
After dividing in two parts, we get
Resulting frustum has height half of the original cone and radius at the top of the frustum equal to half of the base radius of the cone.
Frustum of the cone with radius, R=10 cm and radius r = 5 cm, height =
A smaller cone of radius, r =(R/2) = 5 cm and height =
Therefore, 1:7 is the required ratio.
Note: we have to understand the given problem clearly. If we imagine the problem in geometric structure it is easy to solve. When we divide a cone as mentioned in the problem it looks similar to the below figure.
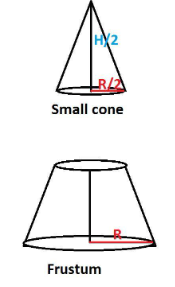
Let the height of the given cone be h cm.
After dividing in two parts, we get
Resulting frustum has height half of the original cone and radius at the top of the frustum equal to half of the base radius of the cone.
Frustum of the cone with radius, R=10 cm and radius r = 5 cm, height =
A smaller cone of radius, r =(R/2) = 5 cm and height =
Therefore, 1:7 is the required ratio.
Note: we have to understand the given problem clearly. If we imagine the problem in geometric structure it is easy to solve. When we divide a cone as mentioned in the problem it looks similar to the below figure.

Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
What is the Full Form of ISI and RAW

A mixture of sand and water can be separated by the class 9 chemistry CBSE

Latitudinal and Longitudinal extent of India is 30 class 9 social science CBSE

Name the three parallel ranges of the Himalayas Describe class 9 social science CBSE

Which among the following has the highest peak in Eastern class 9 social science CBSE

Who is eligible for RTE class 9 social science CBSE




