Question

The front and side view are the same in which figure?
A) Rectangle
B) Square
C) Triangular pyramid
D) Rhombus

Answer 1

Hint:
To solve the question of views, it is highly important that one can visualise shapes and objects from different angles as this will give a clear perception of how they look from any side. If you face difficulty in visualising it, it's always a great idea to draw the shapes and rotate it to view how it looks from different faces.

Complete step by step solution:
A front view is a projection view obtained by drawing perpendiculars from all points on the edges of the part to the plane of projection. The plane of projection upon which the front view is projected is called the frontal plane. A side view is the view of the object when you look at it from a side, that is perpendicular to the normal and the normal pointing in the direction of the front view.
Let’s first draw the front view and side view of a rectangle.

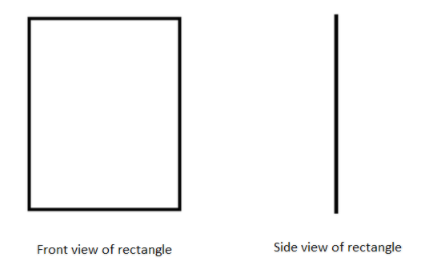
The first image is the front view of the rectangle. When we look at the rectangle from right, we see a straight line as shown in the figure beside the front view. Since, the both are not the same, we discard this option.
Next, let us try to visualise the front view and side view of the square.

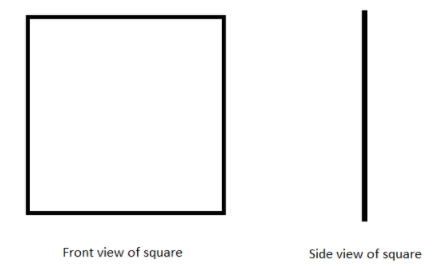
Similar to the rectangle, we can see the front view of the square, and the side view is a straight line. They are also not the same. Hence, we can safely discard squares as an incorrect answer.
Let us move to a triangular pyramid. Triangular pyramid is a 3d-shape. So try to visualise it a bit more carefully. Let us draw the front view and the side view of a triangular pyramid.

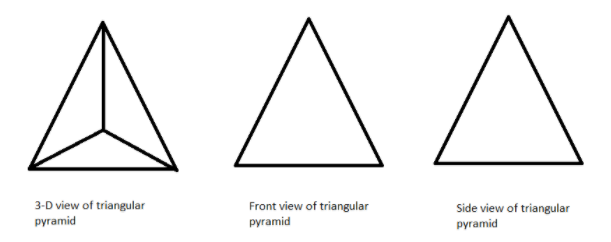
As we can see, the front view and side view of the triangular pyramid are the same, we have found our right answer. But if it is a multiple-choice question, we can not rule out the possibility of our last figure as being one of the correct answers.
So, let us draw and check the front view and side view of rhombus.

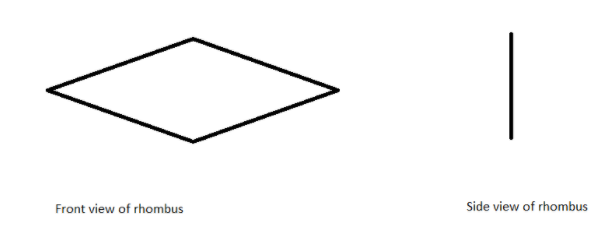
It is clear from the image, that the front view and side view of rhombus are not the same, hence rhombus is the wrong answer.

Thus, the only correct answer is the (C) triangular pyramid.

Note:
Descriptive geometry's primary function is to translate an object in three dimensions into a two-dimensional representation of that object. Each such representation is called a view. To have a look at different views of a figure, rotate it in appropriate angles and look at it from that angle to have an idea of the view.