
The equation of the director circle of the hyperbola
(a)
(b)
(c)
(d)
Answer
535.2k+ views
Hint: First of all, draw a hyperbola and two perpendicular tangents to it and name the point of their intersection as P (h, k) which is the locus of its director circle. Now, write the equation of the tangent of the hyperbola as
Complete step-by-step answer:
In this question, we have to find the director of the circle of the hyperbola
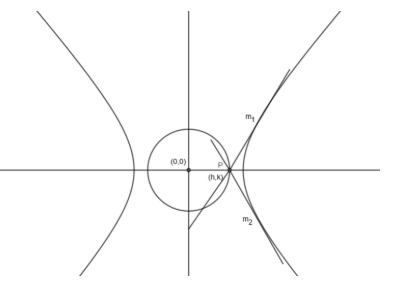
Let the slopes of the two tangents be
We know that the above tangent is passing from P (h, k). So by substituting x = h and y = k, we get,
By squaring both the sides of the above equation, we get,
By using the formula
By rearranging the terms of the above equation, we get,
Above is the quadratic equation in m and we know that the roots of this quadratic equation are
So, we get,
We know that when two lines are perpendicular, the product of their slopes is – 1. As we know that the given tangents are also perpendicular, so we get,
By equating equation (i) and (ii), we get,
By cross multiplying the above equation, we get,
By replacing h by x and k by y, we get,
So, we get the equation of the director circle of the hyperbola
Hence, option (d) is the right answer.
Note: In this question, students must note that this definition of the director circle is true for every curve and not only for the hyperbola. Also, students must remember the equation of the director circle of the same general curves as hyperbola, ellipse, circle, etc. Some students often make a mistake while writing the product of the roots as
Complete step-by-step answer:
In this question, we have to find the director of the circle of the hyperbola

Let the slopes of the two tangents be
We know that the above tangent is passing from P (h, k). So by substituting x = h and y = k, we get,
By squaring both the sides of the above equation, we get,
By using the formula
By rearranging the terms of the above equation, we get,
Above is the quadratic equation in m and we know that the roots of this quadratic equation are
So, we get,
We know that when two lines are perpendicular, the product of their slopes is – 1. As we know that the given tangents are also perpendicular, so we get,
By equating equation (i) and (ii), we get,
By cross multiplying the above equation, we get,
By replacing h by x and k by y, we get,
So, we get the equation of the director circle of the hyperbola
Hence, option (d) is the right answer.
Note: In this question, students must note that this definition of the director circle is true for every curve and not only for the hyperbola. Also, students must remember the equation of the director circle of the same general curves as hyperbola, ellipse, circle, etc. Some students often make a mistake while writing the product of the roots as
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?




