
The difference between outside and inside surfaces of a cylindrical metallic pipe 14 cm long is 44 cm
Answer
512.7k+ views
Hint: First we will first assume that the outer and inner radii of the pipe are
Complete step by step Answer:
We are given that the difference between outside and inside surfaces of a cylindrical metallic pipe 14 cm long is 44 cm
Let us assume that the outer and inner radii of the pipe are
We know that the formula to find the surface area of the cylinder is
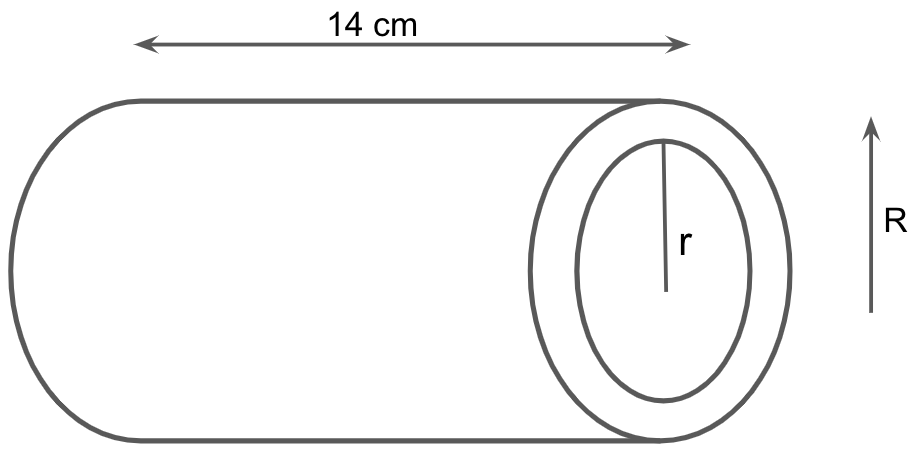
Finding the difference of the outside and inside surface area of a cylindrical pipe using the outer and inner radii, we get
Substituting the value of
Dividing the above equation by 88 on both sides, we get
We know that the formula to find the volume of the cylinder is
Since we are given that the pipe of metal is 99 cm cube, which is the volume of the pipe, by finding the difference of the outside and inside volume of a cylindrical pipe using the outer and inner radii, we get
Substituting the value of
Dividing the above equation by 44 on both sides, we get
Using equation (1) in the above equation, we get
Multiplying the above equation by 2 on both sides, we get
Adding the equation (1) and equation (2), we get
Dividing the above equation by 2 on both sides, we get
Substituting the value of
Thus, the outer radius is
Note: In solving this type of question, the key concept is to assume the inner and outer radii by some distinct variables and also remember the formulae of the circumference of the circle, area of the cylinder, and the volume of the cylinder. After that, the question is really simple if followed properly.
Complete step by step Answer:
We are given that the difference between outside and inside surfaces of a cylindrical metallic pipe 14 cm long is 44 cm
Let us assume that the outer and inner radii of the pipe are
We know that the formula to find the surface area of the cylinder is

Finding the difference of the outside and inside surface area of a cylindrical pipe using the outer and inner radii, we get
Substituting the value of
Dividing the above equation by 88 on both sides, we get
We know that the formula to find the volume of the cylinder is
Since we are given that the pipe of metal is 99 cm cube, which is the volume of the pipe, by finding the difference of the outside and inside volume of a cylindrical pipe using the outer and inner radii, we get
Substituting the value of
Dividing the above equation by 44 on both sides, we get
Using equation (1) in the above equation, we get
Multiplying the above equation by 2 on both sides, we get
Adding the equation (1) and equation (2), we get
Dividing the above equation by 2 on both sides, we get
Substituting the value of
Thus, the outer radius is
Note: In solving this type of question, the key concept is to assume the inner and outer radii by some distinct variables and also remember the formulae of the circumference of the circle, area of the cylinder, and the volume of the cylinder. After that, the question is really simple if followed properly.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
What is the Full Form of ISI and RAW

Which of the following districts of Rajasthan borders class 9 social science CBSE

Difference Between Plant Cell and Animal Cell

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

What is 85 of 500 class 9 maths CBSE




