
The centres of two identical small conducting spheres are 1m apart. They carry charges of opposite kinds and attract each other with a force F. When they are connected by a conducting thin wire they repel each other with a force
A. 1:1
B. 2:1
C. 3:1
D. 4:1
Answer
507.9k+ views
1 likes
Hint: Using Coulomb’s law for electric force, determine the expression for the attractive force between the two spheres in the first case by assuming the charges they carry to be
This stops only after both the spheres have obtained an equal magnitude of charge, which causes them to repel with the force as given in the equation. Obtain the relation for this force and evaluate the resulting equation to obtain the required ratio.
Formula used:
Electric force between two charges:
Complete step-by-step answer:
From Coulomb’s law, we know that the force experienced by two charges
Let us consider the first case, when the two spheres experience an attractive force with respect to each other.
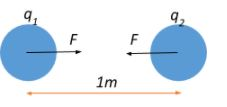
Since they carry opposite charges, let the charges on the spheres be
Now, when the two spheres are connected by a conducting wire, charge begins to flow from one conductor to the other from higher potential (dominantly positively charged conductor) to lower potential (dominantly negatively charged conductor). This flow of charge continues until both the spheres attain the same potential when there is no longer a potential difference between them.
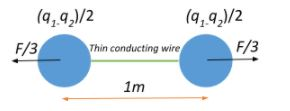
In this case, the charges on both the spheres will be the same, and will be an equivalent distribution of the potential difference (
Since they are like charged, they repel each other with a force given as:
But we are given that
Solving the above equation quadratically:
Taking
Therefore, the correct choice is: C. 3:1
So, the correct answer is “Option C”.
Note: Remember that when the two spheres are connected by the conducting wire, there is always a flow of charge if there exists a potential difference between them such that charges flow from a high potential to a low potential, synonymous to the flow of charge from the positive terminal to the negative terminal of a battery in a circuit since a potential difference sets up an electric field.
Also do not forget that for spherical conductors, charges distribute themselves on the outermost surface so that conducting wire provides only a path for charge transfer and no charge resides on the wire. This is because charges always reside in a way to minimize the potential energy of the system by making the surface an equipotential surface.
This stops only after both the spheres have obtained an equal magnitude of charge, which causes them to repel with the force as given in the equation. Obtain the relation for this force and evaluate the resulting equation to obtain the required ratio.
Formula used:
Electric force between two charges:
Complete step-by-step answer:
From Coulomb’s law, we know that the force experienced by two charges
Let us consider the first case, when the two spheres experience an attractive force with respect to each other.

Since they carry opposite charges, let the charges on the spheres be
Now, when the two spheres are connected by a conducting wire, charge begins to flow from one conductor to the other from higher potential (dominantly positively charged conductor) to lower potential (dominantly negatively charged conductor). This flow of charge continues until both the spheres attain the same potential when there is no longer a potential difference between them.

In this case, the charges on both the spheres will be the same, and will be an equivalent distribution of the potential difference (
Since they are like charged, they repel each other with a force given as:
But we are given that
Solving the above equation quadratically:
Taking
Therefore, the correct choice is: C. 3:1
So, the correct answer is “Option C”.
Note: Remember that when the two spheres are connected by the conducting wire, there is always a flow of charge if there exists a potential difference between them such that charges flow from a high potential to a low potential, synonymous to the flow of charge from the positive terminal to the negative terminal of a battery in a circuit since a potential difference sets up an electric field.
Also do not forget that for spherical conductors, charges distribute themselves on the outermost surface so that conducting wire provides only a path for charge transfer and no charge resides on the wire. This is because charges always reside in a way to minimize the potential energy of the system by making the surface an equipotential surface.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE




