
The area of the trapezium is 1586 sq. m. The length of its parallel sides are 38 cm and 84 cm. Find the distance between them.
Answer
511.5k+ views
Hint: Here, we will draw the diagram using the given information. We will substitute the given values in the formula of area of trapezium. Then we will simplify it to find the distance between the parallel sides by using the area of the trapezium formula. Area of the trapezium is defined as the region covered by the trapezium.
Formula Used:
We will use the formula Area of the Trapezium
Complete step-by-step answer:
First we will draw the diagram of the trapezium based on the given information.
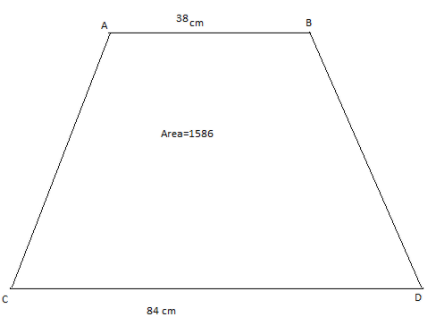
We will substitute the length of the parallel sides and in the formula for area of trapezium.
Substituting
Adding the terms, we get
Dividing 122 by 2, we get
Again dividing 1586 by 61, we get
Therefore, the distance between the parallel sides is 26cm.
Note: We know that the trapezium is also known as trapezoid. A trapezium has two parallel sides and two non-parallel sides. The diagonals of a trapezium bisect each other. The length of the mid-segment is equal to half of the sum of the bases of parallel sides, in a trapezium. Trapezium has a wide number of applications. For example, Roof of a building is in the form of trapezium. If the perpendicular distance between them and the length of the parallel sides are known, then we can find the area of the trapezium.
Formula Used:
We will use the formula Area of the Trapezium
Complete step-by-step answer:
First we will draw the diagram of the trapezium based on the given information.

We will substitute the length of the parallel sides and in the formula for area of trapezium.
Substituting
Adding the terms, we get
Dividing 122 by 2, we get
Again dividing 1586 by 61, we get
Therefore, the distance between the parallel sides is 26cm.
Note: We know that the trapezium is also known as trapezoid. A trapezium has two parallel sides and two non-parallel sides. The diagonals of a trapezium bisect each other. The length of the mid-segment is equal to half of the sum of the bases of parallel sides, in a trapezium. Trapezium has a wide number of applications. For example, Roof of a building is in the form of trapezium. If the perpendicular distance between them and the length of the parallel sides are known, then we can find the area of the trapezium.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Master Class 8 Science: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Trending doubts
Difference Between Plant Cell and Animal Cell

Name 10 Living and Non living things class 9 biology CBSE

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Differentiate between the Western and the Eastern class 9 social science CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

Which places in India experience sunrise first and class 9 social science CBSE




