
The area of the region bounded by
A.
B.
C.
D.
Answer
497.7k+ views
Hint: We plot given lines
Complete step by step answer:
We know that points of intersection are the points of intersection of line with coordinate axes. If the equation of line is
We are given in the question equations of three lines.
We put
We put
We draw line (3) as parallel to the
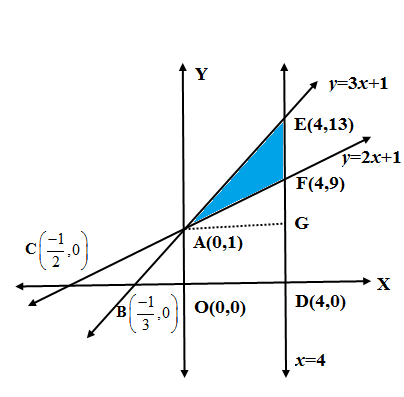
The area enclosed by the lines
So the area of the triangle is
So, the correct answer is “Option D”.
Note: e note that distance (which is always positive) between any two points
Complete step by step answer:
We know that points of intersection are the points of intersection of line with coordinate axes. If the equation of line is
We are given in the question equations of three lines.
We put
We put
We draw line (3) as parallel to the

The area enclosed by the lines
So the area of the triangle is
So, the correct answer is “Option D”.
Note: e note that distance (which is always positive) between any two points
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE

What are the major means of transport Explain each class 12 social science CBSE




