
State the correct statement(s) about the cubic structure of diamond.
A.
B.
C.
D. All
Answer
489.9k+ views
Hint: The structure of diamond is a crystallographic rearrangement of carbon in three dimensional spaces. Diamonds are also called allotropes of carbon which are held together by covalent bonds.
Complete step by step answer:
Diamond is formed by the chemical bonding of carbon atoms. The carbon atoms in diamond are
Let us check the correctness of the given statements one by one.
In the diamond unit cell the carbon atoms are arranged in face centered cubic crystal. The number of atoms that are contributed by the corner atoms in the unit cell is
Out of eight cube corners in the unit cell, four atoms are bonded to an atom within the cube and the other four are bonded to adjacent cubes of the crystal lattice. Hence each carbon atom present at the centre of the tetrahedron in the crystal and four other carbon atoms are present at the corners of the tetrahedron of the face centered cubic crystal.
Let us take a cross section of the cube of the FCC lattice where
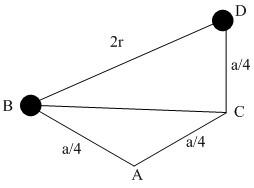
For triangle ABC,
For triangle XYZ,
As
The packing fraction of the lattice is given as
Inserting the value of radius r in the packing fraction relation,
Hence, all the four statements about the cubic structure of diamond are correct.
Note: Please note that in the unit cell of diamond, only
Complete step by step answer:
Diamond is formed by the chemical bonding of carbon atoms. The carbon atoms in diamond are
Let us check the correctness of the given statements one by one.
In the diamond unit cell the carbon atoms are arranged in face centered cubic crystal. The number of atoms that are contributed by the corner atoms in the unit cell is
Out of eight cube corners in the unit cell, four atoms are bonded to an atom within the cube and the other four are bonded to adjacent cubes of the crystal lattice. Hence each carbon atom present at the centre of the tetrahedron in the crystal and four other carbon atoms are present at the corners of the tetrahedron of the face centered cubic crystal.
Let us take a cross section of the cube of the FCC lattice where

For triangle ABC,
For triangle XYZ,
As
The packing fraction of the lattice is given as
Inserting the value of radius r in the packing fraction relation,
Hence, all the four statements about the cubic structure of diamond are correct.
Note: Please note that in the unit cell of diamond, only
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE




