
Solve the following inequality:
Answer
419.7k+ views
2 likes
Hint: We have to find the value of
Complete step by step answer:
Given,
By taking
On solving, we get
On rewriting the above inequality, we get
Simplifying the numerator using the formula:
Adding and subtracting in
As
On further factorising and we get
Taking
As
Taking
Dividing both the sides by
Now we will put all the values of
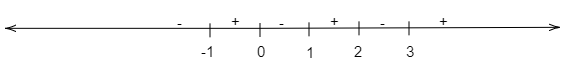
Let’s take any value of
On taking any value greater than
Now taking any number between
Therefore, on taking any value between
Now taking any number between
Therefore, on taking any value between
Now, taking any number between
Therefore, on taking any value between
Now, taking any number between
Therefore, on taking any value between
Now, taking any number less than
Therefore, on taking any number less than
Also, at
Therefore, the interval of
Note:
The solution range of inequality gives us each and every value of
Complete step by step answer:
Given,
By taking
On solving, we get
On rewriting the above inequality, we get
Simplifying the numerator using the formula:
Adding and subtracting in
As
On further factorising and we get
Taking
As
Taking
Dividing both the sides by
Now we will put all the values of

Let’s take any value of
On taking any value greater than
Now taking any number between
Therefore, on taking any value between
Now taking any number between
Therefore, on taking any value between
Now, taking any number between
Therefore, on taking any value between
Now, taking any number between
Therefore, on taking any value between
Now, taking any number less than
Therefore, on taking any number less than
Also, at
Therefore, the interval of
Note:
The solution range of inequality gives us each and every value of
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
What is the Full Form of ISI and RAW

Difference Between Plant Cell and Animal Cell

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

Name 10 Living and Non living things class 9 biology CBSE

Discuss what these phrases mean to you A a yellow wood class 9 english CBSE




