Question

Show that “The tangent at any point of a circle is perpendicular to radius through the point of contact”.

Answer 1

Hint: The tangent of a circle is a straight line which touches the circle at only one point. Here first of all draw the diagram with the radii and the tangent on the circle and follow the properties for the required conditions.

Complete step-by-step answer:

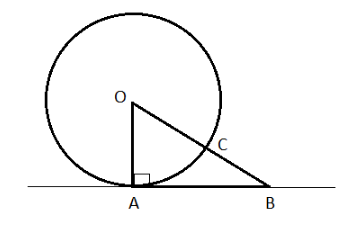
With reference to the above figure-
Since, radii of the same circle are always equal.
 $ OA = OC $ .... (I)
Also, Points O, C and B lie on the same line-segment.
Therefore, it can be written as –
 $ OB = OC + BC $
From the above expression we can say that – OB is greater than OC ( OC being the radius of the circle and B any point on the tangent)
 $ \therefore OB > OC $ .... (II)
From the equation (I) and (II), we can say that –
 $ \Rightarrow OA < OB $
Also, B is an arbitrary point on the tangent as shown in the figure above.
Therefore, OA is the shorter distance than any other line-segment joining O to any point on the tangent.
We also know that the shortest distance of a point from a given line is the perpendicular distance from that line.
Hence, proved – the tangent at any point of a circle is perpendicular to the radius through the point of contact.

Note: The tangent of the circle touches the circle exactly at only one point and is also known as the point of tangency and the tangent of the circle is always perpendicular to the radius. Also, refer to the other tangent theorems.
If two tangents of the same circle are drawn from the common point outside the circle, then the two tangents are congruent to each other.