
Seven resistors are connected as shown in the diagram: The equivalent resistance in ohms of this network between
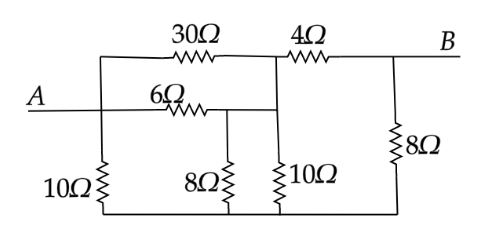
A.
B.
C.
D.

Answer
413.1k+ views
Hint: : Identify the parallel and series connection in the given circuit. First solve for the resistors in parallel connection, then the circuit will become a Wheatstone bridge. Check the condition for a balanced Wheatstone bridge.
Formula used:
Resistors connected in series, equivalence resistance
Resistors connected in parallel, equivalence resistance
Complete step by step answer:
First name the resistances and redraw the circuit diagram.
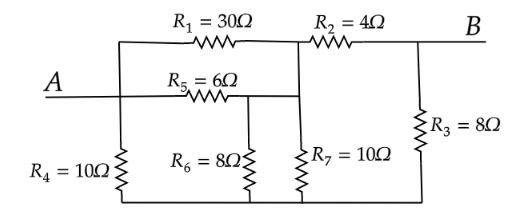
In the above circuit, we have to find the equivalent resistance between the terminal A and B.The resistors
Substitute the values of
The resistors
The equivalent resistor of
Substitute the values of
Now the equivalent circuit of above the circuit is as follows
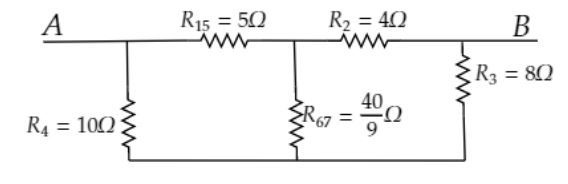
The equivalent circuit of the given circuit is a Wheatstone bridge circuit.
The Wheatstone bridge is balanced because it satisfies the condition
Therefore, no current flows through the resistor
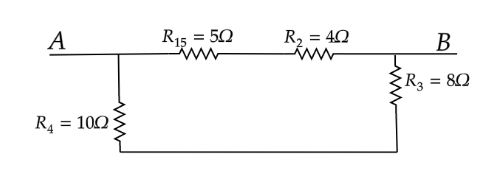
The equivalent resistance of
The equivalent resistance of
Now
The equivalent resistance of
Substitute the values of
The equivalent resistance of the given circuit is
Hence the correct option is A.
Note: Alternative method:Alternatively, we can solve the circuit by drawing an equivalent circuit of the given circuit with some extra consideration as follows.

To solve the above equivalence circuit, Let the current
Apply Kirchhoff's current law at the junction C in the above equivalence circuit.
From the above circuit diagram,
Therefore,
On simplification
Now apply Kirchhoff's current law at junction D in the above equivalence circuit.
From the above circuit diagram,
Therefore,
On simplification
Now solve the two equations (1) and (2) and find the values of
Now calculate the value of
And calculate the value of
Now we have
Substitute the values of
Further calculating
The equivalent resistance of the above equivalence circuit between the two terminals A and B is given by
Substitute all the required values in the above formula
On further simplification
Hence the correct option is (A).
Formula used:
Resistors connected in series, equivalence resistance
Resistors connected in parallel, equivalence resistance
Complete step by step answer:
First name the resistances and redraw the circuit diagram.

In the above circuit, we have to find the equivalent resistance between the terminal A and B.The resistors
Substitute the values of
The resistors
The equivalent resistor of
Substitute the values of
Now the equivalent circuit of above the circuit is as follows

The equivalent circuit of the given circuit is a Wheatstone bridge circuit.
The Wheatstone bridge is balanced because it satisfies the condition
Therefore, no current flows through the resistor

The equivalent resistance of
The equivalent resistance of
Now
The equivalent resistance of
Substitute the values of
The equivalent resistance of the given circuit is
Hence the correct option is A.
Note: Alternative method:Alternatively, we can solve the circuit by drawing an equivalent circuit of the given circuit with some extra consideration as follows.

To solve the above equivalence circuit, Let the current
Apply Kirchhoff's current law at the junction C in the above equivalence circuit.
From the above circuit diagram,
Therefore,
On simplification
Now apply Kirchhoff's current law at junction D in the above equivalence circuit.
From the above circuit diagram,
Therefore,
On simplification
Now solve the two equations (1) and (2) and find the values of
Now calculate the value of
And calculate the value of
Now we have
Substitute the values of
Further calculating
The equivalent resistance of the above equivalence circuit between the two terminals A and B is given by
Substitute all the required values in the above formula
On further simplification
Hence the correct option is (A).
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE




