
Points A and B are situated perpendicular to the axis of a
Answer
495.6k+ views
Hint: Biot-Savart law gives the magnetic field produced due to a current carrying conductor. To find the intensity of the magnetic field at the centre of the current carrying rod of length
Formula Used:
Complete step-by-step solution
Biot-Savart law states that the magnetic field
Mathematically,
Then,
The Biot-Savart law is given on the basis of magnetostatics and it gives the relationship between the current and the magnetic field for any shape of conductor. It is derived from the ampere's circuital law.
Here, given that A and B are at distances X and 3X from its center on opposite sides.
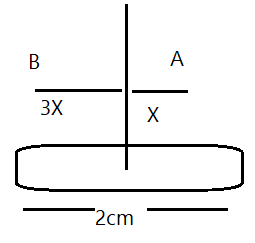
From
Note: From the formula, it is clear that the magnetic field produced depends on the nature of the conductor and the amount of current flowing in the circuit. Note that, we are taking the cross product of the current and the small length, which is why we have a
Formula Used:
Complete step-by-step solution
Biot-Savart law states that the magnetic field
Mathematically,
Then,
The Biot-Savart law is given on the basis of magnetostatics and it gives the relationship between the current and the magnetic field for any shape of conductor. It is derived from the ampere's circuital law.
Here, given that A and B are at distances X and 3X from its center on opposite sides.

From
Note: From the formula, it is clear that the magnetic field produced depends on the nature of the conductor and the amount of current flowing in the circuit. Note that, we are taking the cross product of the current and the small length, which is why we have a
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE




