
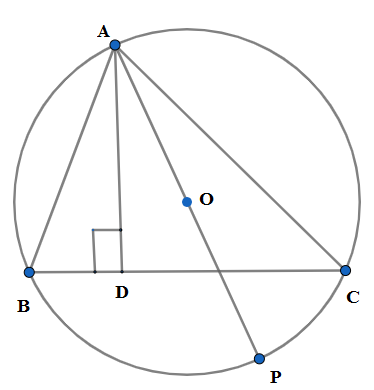
Let triangle ABC have vertices on a circle. Let AD be the altitude and AP be the diameter of the circle. If
A.
B.
C.
D.

Answer
519.3k+ views
Hint: We join BP and CP. We use the theorem that the sum of the angles in a triangle is
Complete step-by-step solution
We are given in the figure a triangle ABC whose vertices are on a circle. AD is the altitude dropped on the side BC and AP is the diameter of the circle. We are further given the measure of angles
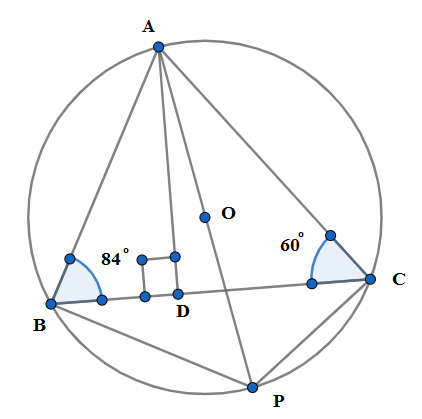
Since AD is the altitude we have
We also have in triangle ADC,
We are given that AP is a diameter which means AP divides the circle into two semi-circles. We know that an arc of semi-circular length always subtends the right angle of measure
Then we have
We know that angles subtended by the same arc have equal measures. Here in the circle arc BP subtends angles
Then we have
So the correct option is D.
Note: We can alternatively solve using the sum of the angles in triangle is
Complete step-by-step solution
We are given in the figure a triangle ABC whose vertices are on a circle. AD is the altitude dropped on the side BC and AP is the diameter of the circle. We are further given the measure of angles

Since AD is the altitude we have
We also have in triangle ADC,
We are given that AP is a diameter which means AP divides the circle into two semi-circles. We know that an arc of semi-circular length always subtends the right angle of measure
Then we have
We know that angles subtended by the same arc have equal measures. Here in the circle arc BP subtends angles
Then we have
So the correct option is D.
Note: We can alternatively solve using the sum of the angles in triangle is
Recently Updated Pages
Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Trending doubts
Why is there a time difference of about 5 hours between class 10 social science CBSE

Indias first jute mill was established in 1854 in A class 10 social science CBSE

Five things I will do to build a great India class 10 english CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

10 examples of evaporation in daily life with explanations




