
Let S be the area of the region enclosed by
A)
B)
C)
D)
Answer
507.6k+ views
Hint: First we have to draw the figure and understand the area
Formula used:
Area enclosed by a curve
Complete step by step answer:
Given that
Let the curve drawn represent
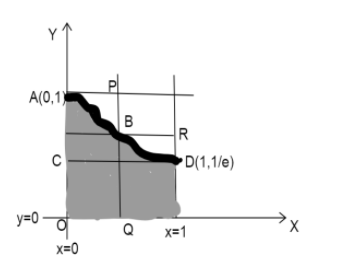
In the figure, the shaded portion represents the area
When
Since
The area of that rectangle will be
Therefore clearly
This implies option A is right.
Also, since
We can consider
Multiplying both sides by
Therefore,
Integrating we get,
Therefore option B is also right.
To show that option C is incorrect,
This implies
From B, we have
Using these two equations we can say C is incorrect.
Moving on, consider two rectangles in the figure OAPQ and QBRS where
Clearly
PQ is drawn parallel to Y axis at the point
Then
Therefore, B is the point
Area of OAPQ
In QBRS,
Therefore, Area of QBRS
So, option D is correct.
Therefore, Option (A), (B) and (D) are correct.
Note:
It is important to identify the graph of the given function and mark the given region. If there is some portion under the X-axis, this area will be negative. So be careful in those cases to split the region if necessary and find the area separately.
Formula used:
Area enclosed by a curve
Complete step by step answer:
Given that
Let the curve drawn represent

In the figure, the shaded portion represents the area
When
Since
The area of that rectangle will be
Therefore clearly
This implies option A is right.
Also, since
We can consider
Multiplying both sides by
Therefore,
Integrating we get,
Therefore option B is also right.
To show that option C is incorrect,
This implies
From B, we have
Using these two equations we can say C is incorrect.
Moving on, consider two rectangles in the figure OAPQ and QBRS where
Clearly
PQ is drawn parallel to Y axis at the point
Then
Therefore, B is the point
Area of OAPQ
In QBRS,
Therefore, Area of QBRS
So, option D is correct.
Therefore, Option (A), (B) and (D) are correct.
Note:
It is important to identify the graph of the given function and mark the given region. If there is some portion under the X-axis, this area will be negative. So be careful in those cases to split the region if necessary and find the area separately.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE

What are the major means of transport Explain each class 12 social science CBSE




