Question

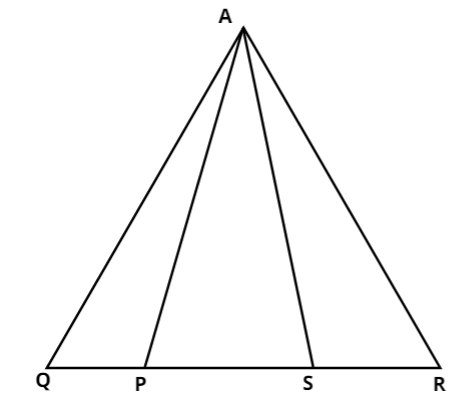
In the figure given above, \[AP \bot l\] that is, \[AP\] is the shortest line segment that can be drawn from \[A\] to the line \[L\]. If \[PR > PQ\], which of the following is true?

A) \[AQ > AR\]
B) \[AR > AQ\]
C) \[AQ = 2AR\]
D) \[AQ = \sqrt 2 AR\]

Answer 1

Hint: At first, we will construct a line which helps to find the answer. Then using the property of congruent triangle and the exterior angle property we will find the required relation between the sides. Among them, we will choose the correct option.

Complete step-by-step answer:
It is given that; \[AP \bot l\] which means, \[AP\] is the shortest line segment that can be drawn from \[A\] to the line \[L\]. Again, \[PR > PQ\].
We have to find the true relation among the given options.
First, we will construct a line which helps us to prove the problem.
Let us cut off \[PS = PQ\] from \[PR\] and we join \[A\] and \[S\].
We know that, two sides and an angle in between the sides of any triangle are equal to the respective sides and angle of another triangle, then these two triangles are said to be the congruent to each other.
Since, \[AP\] is perpendicular to \[QS\], so, \[\angle APQ = \angle APS\], each angle is \[{90^ \circ }\].
Here, in \[\vartriangle APQ\] and \[\vartriangle APS\]
\[\angle APQ = \angle APS\], each angle is \[{90^ \circ }\]
\[AP\] is the common side.
\[PQ = PS\] (from drawing)
So, by SAS condition, \[\vartriangle APQ \cong \vartriangle APS\]
So, we get, \[AP = AS\] and \[\angle AQP = \angle ASP\].........… (1)
From \[\vartriangle ARS\], by exterior angle property we get,
\[\angle ARS < \angle ASP\]..........… (2)
Now, from (1) and (2) we get,
\[\angle ASP > \angle AQP\]
We know that, in any triangle, the largest side and largest angle are opposite one another and the smallest side and smallest angle are opposite one another.
So, we have, \[AR > AQ\]

Hence, the correct option is B.

Note: We know that, two sides and an angle in between the sides of any triangle are equal to the respective sides and angle of another triangle, then these two triangles are said to be the congruent to each other.
We know that, in any triangle, the largest side and largest angle are opposite one another and the smallest side and smallest angle are opposite one another.