Answer
392.4k+ views
Hint: Here we are given that $AD \bot BC$. So we got two right angled triangles. So using the Pythagoras theorem in the $\Delta ABD$
$A{B^2} = A{D^2} + B{D^2}$ and in the triangle $ADC$, we get that
$A{C^2} = A{D^2} + D{C^2}$
Complete step-by-step answer:
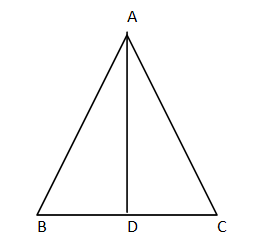
In the given figure in the triangle $ABC$ where $\angle B < 90^\circ $
To prove: $A{C^2} = A{B^2} + B{C^2} - 2BC.BD$
And$AD$ is the product such that$AD \bot BC$. Therefore we have got two right angled triangles.
Here we get that $AD \bot BC$.
So now in$\Delta ABD$
$AD \bot BC$ so $\angle ADB = 90^\circ $
So we can use the Pythagoras theorem where
${({\text{hypotenuse)}}^2} = {({\text{base)}}^2} + {{\text{(perpendicular)}}^2}$
In$\Delta ABD$
$A{B^2} = A{D^2} + B{D^2}$$ - - - - - (1)$
In $\Delta ADC$
$AD \bot BC$
So $\angle ADC = 90^\circ $
Hence we get that
$A{C^2} = A{D^2} + D{C^2}$$ - - - - (2)$
Now we substitute $DC = BC - BD$ and also the value got from (1)
So putting these in the equation (2), we get
$A{C^2} = A{B^2} - B{D^2} + B{C^2} + B{D^2} - 2BC.BD$
So we get that
$A{C^2} = A{B^2} + B{C^2} - 2BC.BD$
Hence proved.
Note: In the right angled triangle we use the Pythagoras theorem. In that right angled triangle, the side opposite to the $90^\circ $ is considered as hypotenuse of that triangle and we can use Pythagoras theorem as ${({\text{hypotenuse)}}^2} = {({\text{base)}}^2} + {{\text{(perpendicular)}}^2}$
$A{B^2} = A{D^2} + B{D^2}$ and in the triangle $ADC$, we get that
$A{C^2} = A{D^2} + D{C^2}$
Complete step-by-step answer:

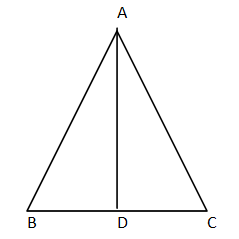
In the given figure in the triangle $ABC$ where $\angle B < 90^\circ $
To prove: $A{C^2} = A{B^2} + B{C^2} - 2BC.BD$
And$AD$ is the product such that$AD \bot BC$. Therefore we have got two right angled triangles.
Here we get that $AD \bot BC$.
So now in$\Delta ABD$
$AD \bot BC$ so $\angle ADB = 90^\circ $
So we can use the Pythagoras theorem where
${({\text{hypotenuse)}}^2} = {({\text{base)}}^2} + {{\text{(perpendicular)}}^2}$
In$\Delta ABD$
$A{B^2} = A{D^2} + B{D^2}$$ - - - - - (1)$
In $\Delta ADC$
$AD \bot BC$
So $\angle ADC = 90^\circ $
Hence we get that
$A{C^2} = A{D^2} + D{C^2}$$ - - - - (2)$
Now we substitute $DC = BC - BD$ and also the value got from (1)
So putting these in the equation (2), we get
$A{C^2} = A{B^2} - B{D^2} + B{C^2} + B{D^2} - 2BC.BD$
So we get that
$A{C^2} = A{B^2} + B{C^2} - 2BC.BD$
Hence proved.
Note: In the right angled triangle we use the Pythagoras theorem. In that right angled triangle, the side opposite to the $90^\circ $ is considered as hypotenuse of that triangle and we can use Pythagoras theorem as ${({\text{hypotenuse)}}^2} = {({\text{base)}}^2} + {{\text{(perpendicular)}}^2}$
Recently Updated Pages
The branch of science which deals with nature and natural class 10 physics CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Define absolute refractive index of a medium

Find out what do the algal bloom and redtides sign class 10 biology CBSE

Prove that the function fleft x right xn is continuous class 12 maths CBSE

Find the values of other five trigonometric functions class 10 maths CBSE

Trending doubts
Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

How fast is 60 miles per hour in kilometres per ho class 10 maths CBSE

What organs are located on the left side of your body class 11 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

How do you solve x2 11x + 28 0 using the quadratic class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Which are the major cities located on the river Ga class 10 social science CBSE

What is BLO What is the full form of BLO class 8 social science CBSE