
In the circuit shown, the total current supplied by the battery is
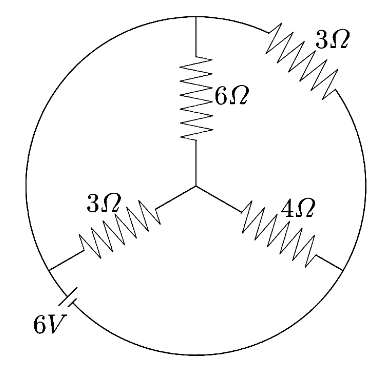
A.
B.
C.
D.

Answer
437.4k+ views
Hint: We can find the total current supplied by the battery using Ohm’s Law which states that voltage of a battery is the product of the current supplied and the total resistance. To find the total resistance, we need to rearrange the given circuit to understand the series and parallel connections.
Formula used:
Series connection of resistors
Parallel connection of resistors
Ohm’s Law
Complete step by step answer:
Let us note down the given data,
Now, let us name the nodes to understand the series and parallel connections of resistors as shown below,
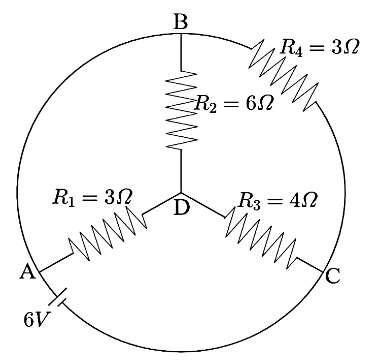
Now, from the figure above, we can see that between the points
Thus, we can see that the resistors
Now, the combination of
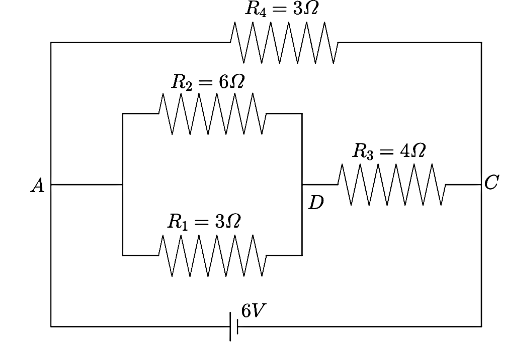
Now, we can see from the above figure that the resistance
Substituting the given values,
Taking the reciprocal on both sides,
Now, the circuit can be drawn as shown below,
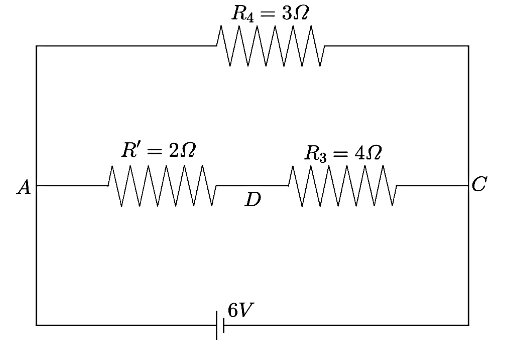
Now, the resistances
Substituting the given values,
Now, the circuit can be redrawn as shown below,
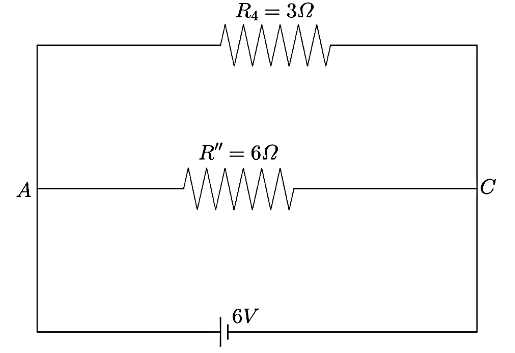
Now, the resistances
Substituting the given values,
Taking the reciprocal on both sides,
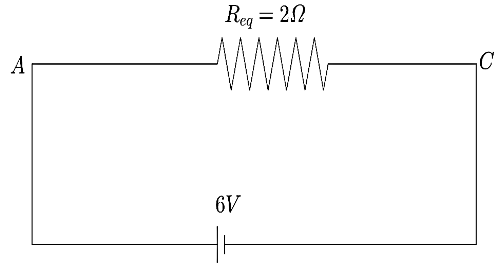
This is the total resistance of the circuit. Now, the total current can be calculated using the Ohm’s Law
Substituting the given values,
Hence, the correct answer is option C.
Note: We should note that in this type of problem, we should always start by naming the junctions in the circuit. While naming, if there is no other component other than a simple wire between the two points, then those two points are considered to be the same. We should remember that resistors are said to be in parallel when they are connected between the same two points, and they are said to be in series when they are connected in a line.
Formula used:
Series connection of resistors
Parallel connection of resistors
Ohm’s Law
Complete step by step answer:
Let us note down the given data,
Now, let us name the nodes to understand the series and parallel connections of resistors as shown below,

Now, from the figure above, we can see that between the points
Thus, we can see that the resistors
Now, the combination of

Now, we can see from the above figure that the resistance
Substituting the given values,
Taking the reciprocal on both sides,
Now, the circuit can be drawn as shown below,

Now, the resistances
Substituting the given values,
Now, the circuit can be redrawn as shown below,

Now, the resistances
Substituting the given values,
Taking the reciprocal on both sides,

This is the total resistance of the circuit. Now, the total current can be calculated using the Ohm’s Law
Substituting the given values,
Hence, the correct answer is option C.
Note: We should note that in this type of problem, we should always start by naming the junctions in the circuit. While naming, if there is no other component other than a simple wire between the two points, then those two points are considered to be the same. We should remember that resistors are said to be in parallel when they are connected between the same two points, and they are said to be in series when they are connected in a line.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE




