
In the adjoining figure, the radius of the inner circles, if other circles are of m units is
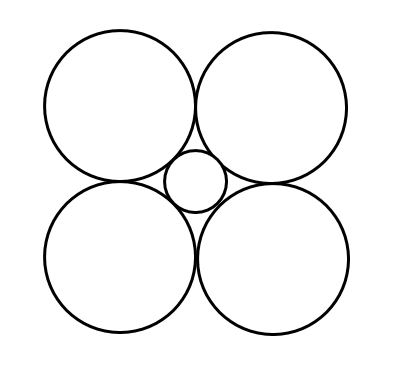
A.
B.
C.
D.

Answer
516.3k+ views
Hint: First of all, redraw the figure of this problem by joining all the centres of the outer circles to form a square of side length
Complete step by step solution:
If we join all the centres of the four outer circles then it forms a square of side
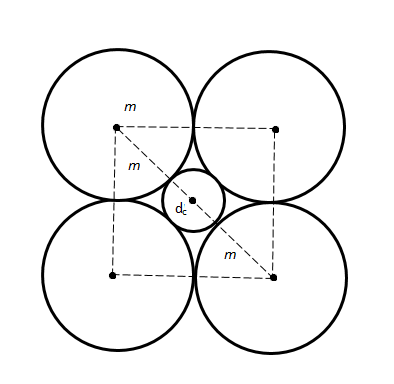
We know that the diagonal of the square of side
Thus, the length of the diagonal formed is
Clearly from the figure, the diagonal of the square passes through the centre of the inner circle and the length of the diagonal is equal to the sum of radius of the two outer circles and the diameter of the inner circle.
Let
So, we have
We know the radius of a circle is equal to half of the length of its diameter.
Hence, the radius of inner circle
Thus, the correct option is A.
Note: The diagonal of the square of side
Complete step by step solution:
If we join all the centres of the four outer circles then it forms a square of side

We know that the diagonal of the square of side
Thus, the length of the diagonal formed is
Clearly from the figure, the diagonal of the square passes through the centre of the inner circle and the length of the diagonal is equal to the sum of radius of the two outer circles and the diameter of the inner circle.
Let
So, we have
We know the radius of a circle is equal to half of the length of its diameter.
Hence, the radius of inner circle
Thus, the correct option is A.
Note: The diagonal of the square of side
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
What is the Full Form of ISI and RAW

Which of the following districts of Rajasthan borders class 9 social science CBSE

Difference Between Plant Cell and Animal Cell

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

What is 85 of 500 class 9 maths CBSE




