
In a town of 10,000 families it was found that 40% families buy newspaper A, 20% families buy newspaper B and 10% families buy newspaper C. 5%families buy A and B, 3% buy B and C and 4% buy A and C. If 2% families buy all the three newspaper, find the number of families which buy (1) A only (2) B only (3) none of A, B, C
(a) (1) 3000, (2) 1800, (3) 4600
(b) (1) 3300, (2) 1400, (3) 4000
(c) (1) 3500, (2) 1600, (3) 3800
(d) none
Answer
453.9k+ views
7 likes
Hint: In this question, we first need to draw the venn diagram using the given conditions so that we get a clear picture of what we have to find. Then subtracting the number of families reading A & C , A & B and then adding the families reading all three gives the value of A only. In the same way subtract families reading B & C, B & A and then add those reading three to get the value of B only. Now, subtracting the whole things inside the venn diagram from 10000 gives the families reading none.
Complete step-by-step answer:
Now, the total number of families in the town are 10,000
Now, given that 40% of them buy newspaper A
Thus, 4000 families read A
Now, given that 20% of them buy newspaper B
Thus, 2000 families buy B
Now, given that 10% of them buy newspaper C
Thus, 1000 families buy C
Now, given that 5% of them buy newspaper A & B
Thus, 500 families buy both A & B.
Now, given that 3% of them buy newspaper B & C
Thus, 300 families buy both B & C.
Now, given that 4% of them buy newspaper A & C
Thus, 400 families buy both A & C.
Now, given that 2% of them buy newspaper A & B & C
Thus, 200 families buy all A & B & C
Now, let us draw the venn diagram
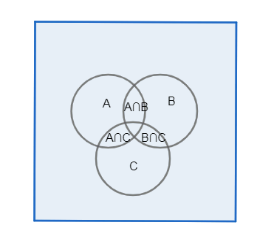
(1) Now, let us find the number of families that buy only A
Now, on substituting the respective values we get,
Now, on further simplification we get,
Thus, 3300 families buy only A
(2) Now, let us find the number of families that buy only B
Now, on substituting the respective values we get,
Now, on further simplification we get,
Thus, 1400 families buy only B
(3) Let us now find the families that buy none of A, B, C
Now, on substituting the respective values we get,
Now, on further simplification we get,
Now, this can be further written as
Thus, 4000 families buy none of the newspaper
Hence, the correct option is (b).
Note: It is important to note that we need to draw the venn diagram first and then add or subtract the things accordingly by observing the venn diagram. Here, when we find only A after subtracting the things common to A & B, A & C we need to add
It is also important to note that while doing the calculations we should not neglect any of the terms or consider it multiple times because it changes the result completely.
Complete step-by-step answer:
Now, the total number of families in the town are 10,000
Now, given that 40% of them buy newspaper A
Thus, 4000 families read A
Now, given that 20% of them buy newspaper B
Thus, 2000 families buy B
Now, given that 10% of them buy newspaper C
Thus, 1000 families buy C
Now, given that 5% of them buy newspaper A & B
Thus, 500 families buy both A & B.
Now, given that 3% of them buy newspaper B & C
Thus, 300 families buy both B & C.
Now, given that 4% of them buy newspaper A & C
Thus, 400 families buy both A & C.
Now, given that 2% of them buy newspaper A & B & C
Thus, 200 families buy all A & B & C
Now, let us draw the venn diagram

(1) Now, let us find the number of families that buy only A
Now, on substituting the respective values we get,
Now, on further simplification we get,
Thus, 3300 families buy only A
(2) Now, let us find the number of families that buy only B
Now, on substituting the respective values we get,
Now, on further simplification we get,
Thus, 1400 families buy only B
(3) Let us now find the families that buy none of A, B, C
Now, on substituting the respective values we get,
Now, on further simplification we get,
Now, this can be further written as
Thus, 4000 families buy none of the newspaper
Hence, the correct option is (b).
Note: It is important to note that we need to draw the venn diagram first and then add or subtract the things accordingly by observing the venn diagram. Here, when we find only A after subtracting the things common to A & B, A & C we need to add
It is also important to note that while doing the calculations we should not neglect any of the terms or consider it multiple times because it changes the result completely.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
What is the Full Form of ISI and RAW

Difference Between Plant Cell and Animal Cell

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

Name 10 Living and Non living things class 9 biology CBSE

Discuss what these phrases mean to you A a yellow wood class 9 english CBSE




