
How do you find trigonometric ratios of 30, 45, and 60 degrees?
Answer
488.7k+ views
1 likes
Hint: Right-angled triangle in an equilateral triangle (which is formed by dropping altitude from any vertex to its opposite side) and isosceles right-angled triangle are considered to be special right triangles. From these triangles, we can find the trigonometric ratios of basic angles. Trigonometric ratios are the ratios of the sides of some standard triangles like a special right triangle. In a triangle, according to the Pythagorean theorem, the sum of squares of the length of two adjacent sides is equal to the square of the length of the opposite side.
Complete step-by-step solution:
As per the given question, we have trigonometric ratios of 30, 45, and 60 degrees. Both
The
Now, let’s consider the equilateral triangle which is shown below:
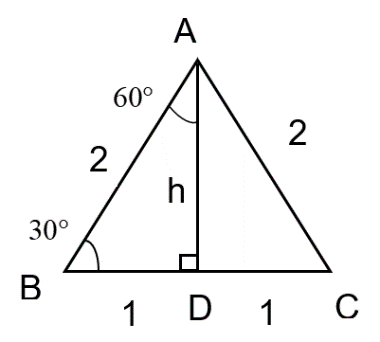
Using Pythagorean theorem,
cosec
Therefore, the trigonometric ratios of
Now, let’s consider the isosceles right triangle to find trigonometric ratios of
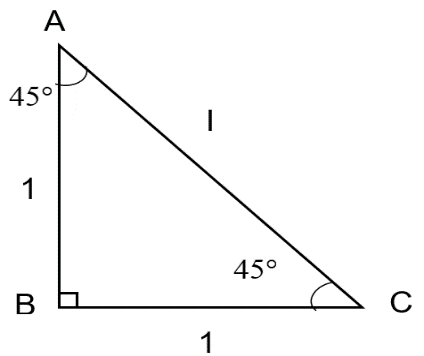
Using Pythagorean theorem, length
Therefore, the trigonometric ratios of
Note: While finding the trigonometric ratios of a triangle, if we have sine, cosine, and tangent values, then we can calculate the cosecant, secant, and cotangent of the triangle. And we know that for
Complete step-by-step solution:
As per the given question, we have trigonometric ratios of 30, 45, and 60 degrees. Both
The
Now, let’s consider the equilateral triangle which is shown below:

Using Pythagorean theorem,
cosec
Therefore, the trigonometric ratios of
Now, let’s consider the isosceles right triangle to find trigonometric ratios of

Using Pythagorean theorem, length
Therefore, the trigonometric ratios of
Note: While finding the trigonometric ratios of a triangle, if we have sine, cosine, and tangent values, then we can calculate the cosecant, secant, and cotangent of the triangle. And we know that for
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Which tributary of Indus originates from Himachal Pradesh class 10 social science CBSE

Distinguish between ordinary light and laser light class 10 physics CBSE

What is the past tense of read class 10 english CBSE

The minimum age prescribed for election as a member class 10 social science CBSE

Two tankers contain 850 litres and 680 litres of petrol class 10 maths CBSE




