
Find the largest number that divides 2053 and 967 and leaves a remainder of 5 and 7 respectively.
Answer
442.5k+ views
3 likes
Hint: H.C.F can be defined as the highest number which divides the given numbers and gives remainder zero. Apply this rule to get an answer.
Let us suppose that number is ‘N’ which divides 2053 and 967 and leaves a remainder 5 and 7 respectively.
Now, the first number is given as 2053 which is divided by N and given remainder 5. Now, if we subtract 5 from 2053, we get 2053 – 5 = 2048 which will give remainder zero if we divide it by N now.
For second number 967, if we divide it by N, we get remainder 7 which means if we divide 967 – 7 =960 by N, we will get remainder zero.
Hence, now one can rewrite the question as evaluating the number which divides 2048 and 960 and gives remainder 0.
Now, we can relate that number N should be the highest common factor of 2048 and 960 which will divide the given numbers and the remainder will be zero.
So, let us find H.C.F by prime factorization method.
Prime factorization of 960 can be given as;
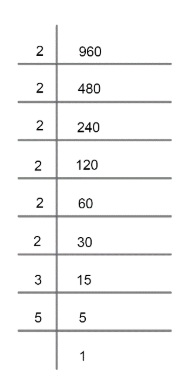
Hence,
Now, factoring 2048 as
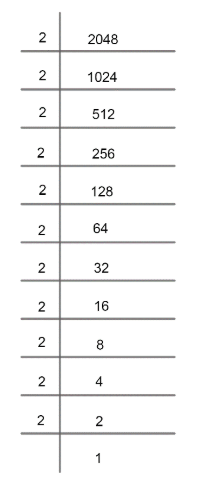
Hence, 2048can be expressed as;
Now, as we know the prime factorization method that the terms common in the factorization of numbers will give the HCF.
Hence, common factors in 960 and 2048;
H.C.F = 64
Hence, number N is 64 which will give remainders 5 and 7 on dividing 2053 and 967 respectively.
Note: One can go wrong after subtraction of remainders from the given numbers. He/she may go for calculation of L.C.M (Least common multiple) in place of H.C.F which will give the wrong solution.
Hence, terms H.C.F and L.C.M should be very clear for this question.
One can miss any prime factor from the expression of prime factorization of 960 and 2048.
Let us suppose that number is ‘N’ which divides 2053 and 967 and leaves a remainder 5 and 7 respectively.
Now, the first number is given as 2053 which is divided by N and given remainder 5. Now, if we subtract 5 from 2053, we get 2053 – 5 = 2048 which will give remainder zero if we divide it by N now.
For second number 967, if we divide it by N, we get remainder 7 which means if we divide 967 – 7 =960 by N, we will get remainder zero.
Hence, now one can rewrite the question as evaluating the number which divides 2048 and 960 and gives remainder 0.
Now, we can relate that number N should be the highest common factor of 2048 and 960 which will divide the given numbers and the remainder will be zero.
So, let us find H.C.F by prime factorization method.
Prime factorization of 960 can be given as;

Hence,
Now, factoring 2048 as

Hence, 2048can be expressed as;
Now, as we know the prime factorization method that the terms common in the factorization of numbers will give the HCF.
Hence, common factors in 960 and 2048;
H.C.F = 64
Hence, number N is 64 which will give remainders 5 and 7 on dividing 2053 and 967 respectively.
Note: One can go wrong after subtraction of remainders from the given numbers. He/she may go for calculation of L.C.M (Least common multiple) in place of H.C.F which will give the wrong solution.
Hence, terms H.C.F and L.C.M should be very clear for this question.
One can miss any prime factor from the expression of prime factorization of 960 and 2048.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
What is the Full Form of ISI and RAW

Difference Between Plant Cell and Animal Cell

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

What is 85 of 500 class 9 maths CBSE

Name 10 Living and Non living things class 9 biology CBSE




