
How do you find the coterminal with the angle
Answer
471.6k+ views
Hint: We are given angle as
Complete step by step answer:
We are given as
Firstly we will understand that the negative sign symbolic the orientation.
Now we work on our problem, we start by understanding what are coterminal angles.
coterminal angles are angles in standard position that mean angles with the initial side on the positive x-axis and have the common terminal side.
For example
If we sketch them on graph, we get better understanding
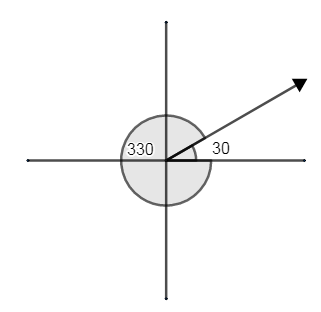
Here
So, they are coterminal angles by definition.
They can be more than 1 coterminal angle for any given angle, like for 30, apart from -330 degree, we have 390degree.

In general, if
Now, we work on our problem, we have
We start by considering
As we know that for angle
So, for
Its coterminal angle is
We put
So, we get a coterminal angle with angle
Note:
Depending upon ‘n’, we can have many other coterminal angle also graphically
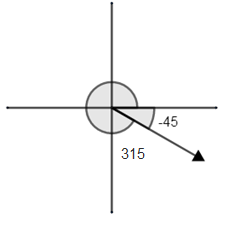
So, for
Remember that positive or negative sign in the angle is regard to the orientation, if we measure angle in clockwise direction this it is negative, if we measure anti-clock wise then it is positive also graph way to find coterminal angle is also strong as we can clearly see whether condition of being coterminal angle are satisfied or not.
Complete step by step answer:
We are given as
Firstly we will understand that the negative sign symbolic the orientation.
Now we work on our problem, we start by understanding what are coterminal angles.
coterminal angles are angles in standard position that mean angles with the initial side on the positive x-axis and have the common terminal side.
For example
If we sketch them on graph, we get better understanding

Here
So, they are coterminal angles by definition.
They can be more than 1 coterminal angle for any given angle, like for 30, apart from -330 degree, we have 390degree.

In general, if
Now, we work on our problem, we have
We start by considering
As we know that for angle
So, for
Its coterminal angle is
We put
So, we get a coterminal angle with angle
Note:
Depending upon ‘n’, we can have many other coterminal angle also graphically

So, for
Remember that positive or negative sign in the angle is regard to the orientation, if we measure angle in clockwise direction this it is negative, if we measure anti-clock wise then it is positive also graph way to find coterminal angle is also strong as we can clearly see whether condition of being coterminal angle are satisfied or not.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
What is the Full Form of ISI and RAW

Difference Between Plant Cell and Animal Cell

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

Name 10 Living and Non living things class 9 biology CBSE

Discuss what these phrases mean to you A a yellow wood class 9 english CBSE





