
Express 1.2 radian in degree measure.
Answer
531.6k+ views
Hint:The given problem is related to unit conversion. To convert from radian to degree we have to multiply by either
Complete step-by-step answer:
In the question we are given an angle in radian which is 1.2 rad and we have to convert it into degrees.
Before proceeding we will first briefly say something about radian.
The radian is an S.I. unit for measuring angles and is the standard unit of angular measure used in areas of mathematics. The length of an arc of unit circle is numerically equal to the measurement in radians of the angle that it subtends; one radian is just under 57.3 degrees.
Radian describes the plain angle subtended by a circular arc as the length of arc divided by radius of the arc. One radian is the angle subtended at the center of a circle by an arc that is equal in length to the magnitude in radians of such a subtend angle is equal to the ratio of the arc length to the radius of circle; that is
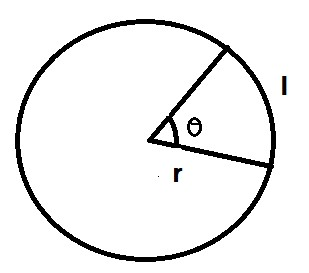
Conversely, the length of the enclosed arc is equal to the radius multiplied by the magnitude of the angle in radians that is
We are given the angle value as 1.2 radian. So, to change it into a degree we have to multiply by
So, we can write it as,
Hence, the value is
Note: Students should think before multiplying by either
Complete step-by-step answer:
In the question we are given an angle in radian which is 1.2 rad and we have to convert it into degrees.
Before proceeding we will first briefly say something about radian.
The radian is an S.I. unit for measuring angles and is the standard unit of angular measure used in areas of mathematics. The length of an arc of unit circle is numerically equal to the measurement in radians of the angle that it subtends; one radian is just under 57.3 degrees.
Radian describes the plain angle subtended by a circular arc as the length of arc divided by radius of the arc. One radian is the angle subtended at the center of a circle by an arc that is equal in length to the magnitude in radians of such a subtend angle is equal to the ratio of the arc length to the radius of circle; that is

Conversely, the length of the enclosed arc is equal to the radius multiplied by the magnitude of the angle in radians that is
We are given the angle value as 1.2 radian. So, to change it into a degree we have to multiply by
So, we can write it as,
Hence, the value is
Note: Students should think before multiplying by either
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE




