
Draw circuit diagram of R-C phase-shift oscillator. Find frequency of oscillation if
Answer
502.2k+ views
1 likes
Hint: RC circuit is a combination of an amplifier and RC feedback network. A typical R-C circuit has a combination of three resistors and capacitors. Use these details to draw the circuit diagram of R-C phase-shift oscillator. Then, to find the frequency of oscillation use the formula for frequency of R-C phase-shift oscillator. Substitute the given values and find the frequency of oscillation.
Formula used:
Complete answer:
R-C phase shift oscillators use a combination of an amplifier and an RC feedback network to produce output oscillations due to the phase shift between the stages.
R-C phase oscillator is shown below.
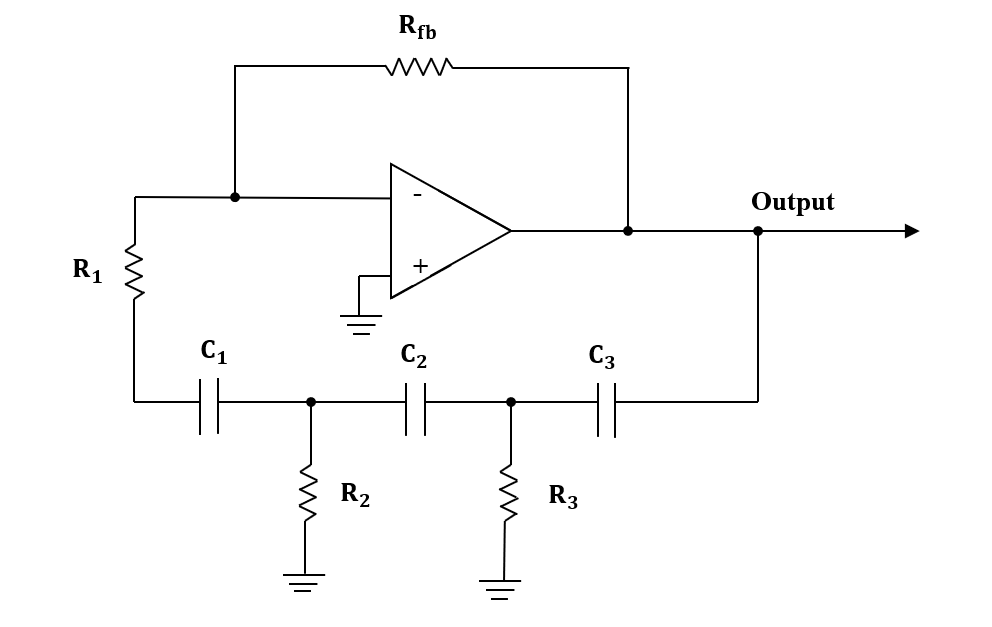
Given:
Frequency of a R-C phase-shift oscillator is given by,
Where, R is the feedback resistance
C is the feedback capacitance
N is the number of RC combinations
Here, we have three RC combinations. So, N= 3.
Substituting values in the equation. (1) we get,
Hence, the frequency of oscillation is 1 Hz.
Note:
If we want to vary the frequency then this can be done by varying one or more of the resistors or the capacitors in the phase-shift network. RC phase shift oscillators are generally used for low frequencies. The output which we get from this RC circuit is sinusoidal in nature. This circuit is not expensive and gives excellent frequency stability. Disadvantage of this circuit is that the output from this circuit is very small because of smaller feedback.
Formula used:
Complete answer:
R-C phase shift oscillators use a combination of an amplifier and an RC feedback network to produce output oscillations due to the phase shift between the stages.
R-C phase oscillator is shown below.

Given:
Frequency of a R-C phase-shift oscillator is given by,
Where, R is the feedback resistance
C is the feedback capacitance
N is the number of RC combinations
Here, we have three RC combinations. So, N= 3.
Substituting values in the equation. (1) we get,
Hence, the frequency of oscillation is 1 Hz.
Note:
If we want to vary the frequency then this can be done by varying one or more of the resistors or the capacitors in the phase-shift network. RC phase shift oscillators are generally used for low frequencies. The output which we get from this RC circuit is sinusoidal in nature. This circuit is not expensive and gives excellent frequency stability. Disadvantage of this circuit is that the output from this circuit is very small because of smaller feedback.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




