
Define the circle segment and sector of a circle.
Answer
514.2k+ views
1 likes
Hint: Segment of a circle is the region bounded by a chord and the arc subtended by the chord.
Sector of the circle looks like a pizza slice.
Complete step by step solution: Segment is the region of a circle bounded by chord and an arc.
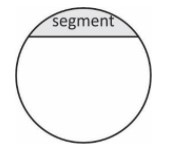
The segments are explained in two parts:
As we show in the above diagram a line divides the circle in two parts in which the biggest part of the circle is called the major segment.
Or the lower part or portion is known as a minor segment.
We also calculate the area of segment:
The area of the segment is equal to area of sector minus of area of triangular piece.
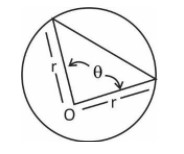

Sector of Circle
The shaded region is the sector of circle.

A sector is created by the central angle formed with two radii and it includes the area inside the circle from that center point to the circle itself. The portion of the circle's circumference bounded by the radii, the arc, is part of the sector.
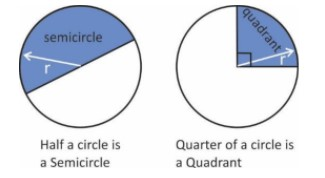
Common Sectors
The quadrant and semicircle are two special types of sector:
Area of sector
Where
Note: A circle has an angle of
Sector of the circle looks like a pizza slice.
Complete step by step solution: Segment is the region of a circle bounded by chord and an arc.

The segments are explained in two parts:
As we show in the above diagram a line divides the circle in two parts in which the biggest part of the circle is called the major segment.
Or the lower part or portion is known as a minor segment.
We also calculate the area of segment:
The area of the segment is equal to area of sector minus of area of triangular piece.


Sector of Circle
The shaded region is the sector of circle.

A sector is created by the central angle formed with two radii and it includes the area inside the circle from that center point to the circle itself. The portion of the circle's circumference bounded by the radii, the arc, is part of the sector.
Common Sectors
The quadrant and semicircle are two special types of sector:

Area of sector
Where
Note: A circle has an angle of
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

How many ounces are in 500 mL class 8 maths CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE

Name the states through which the Tropic of Cancer class 8 social science CBSE

Explain land use pattern in India and why has the land class 8 social science CBSE

One cusec is equal to how many liters class 8 maths CBSE




