
An infinite nonconducting sheet has a surface charge density
A.
B.
C.
D.
Answer
486.6k+ views
Hint: Potential of a charge conducting field is the product of the electric field and the distance between the parallel plates of the charged conductors. And the electric field on an infinite sheet is the ratio of its charge density to the relative permittivity.
Formula Used:
Where,
Where,
Complete step by step answer:Observe the diagram
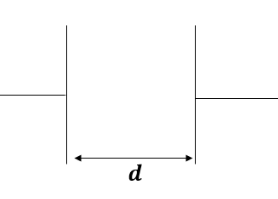
We know that, for an infinite charged sheet
and
where,
Substituting the value of
By rearranging it, we get
It is given to us that,
Substituting these values in the above equation, we get
by simplifying it we get
Therefore from the above explanation the correct option is (B)
Note:Any surface over which the potential is constant is called an equipotential surface. In other words, the potential difference between any two points to an equipotential surface is zero. For this question, we considered the relativity of free space and it was not mentioned that there is any dielectric between the plates. But it is not always necessary. Sometimes, you might have a dielectric between the plates. Then the formula of electric field will be
Where,
Formula Used:
Where,
Where,
Complete step by step answer:Observe the diagram

We know that, for an infinite charged sheet
and
where,
Substituting the value of
By rearranging it, we get
It is given to us that,
Substituting these values in the above equation, we get
by simplifying it we get
Therefore from the above explanation the correct option is (B)
Note:Any surface over which the potential is constant is called an equipotential surface. In other words, the potential difference between any two points to an equipotential surface is zero. For this question, we considered the relativity of free space and it was not mentioned that there is any dielectric between the plates. But it is not always necessary. Sometimes, you might have a dielectric between the plates. Then the formula of electric field will be
Where,
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Why is insulin not administered orally to a diabetic class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Define Vant Hoff factor How is it related to the degree class 12 chemistry CBSE

The first microscope was invented by A Leeuwenhoek class 12 biology CBSE

The current flowing through the resistor in a series class 12 physics CBSE

Name the part of the flower which the tassels of the class 12 biology CBSE




