
A wire of resistance
A. 12
B. 6
C. 4
D. 9
Answer
507k+ views
Hint: Draw an equivalent circuit diagram of the given situation to determine the resistance across any two vertices of the equilateral triangle. Use the formula for the equivalent resistance for the resistors in series and parallel.
Formula used:
The equivalent resistance of the two resistors connected in series is
Here,
The equivalent resistance of the two resistors connected in parallel is
Here,
Complete step by step answer:
The equilateral triangle is a triangle in which all of its three edges are of the same length.
The total resistance across any wire is directly proportional to the length of the wire.
The total resistance of the given wire is
The given wire is bent to form an equilateral triangle. Hence, the total resistance
Hence, the resistance through each edge of the equilateral triangle is
Now, calculate the resistance across any two vertices of the equilateral triangle.
The resistance across any two vertices of the equilateral triangle should be different as across any two vertices of the equilateral triangle two resistances are in series.
The diagram representing the resistances across 3 edges of the equilateral triangle and its equivalent circuit is as follows:
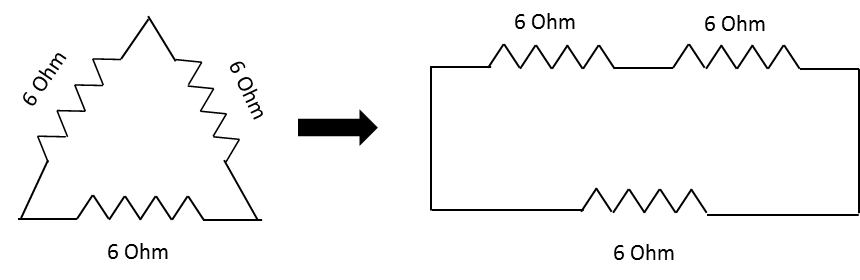
From the above diagram, it is clear that when a wire with resistance is bent into a equilateral triangle, its resistance divides equally in three edges of triangle and the its equivalent circuit across two vertices of the triangle contains resistance of the two edges of the triangle in series and resistance of one edge in parallel to them.
Let the two resistors in series be labeled
Calculate the equivalent resistance
Substitute
Hence, the equivalent resistance across the two parallel resistors is
Now, calculate the equivalent
Rewrite equation (2) for the equivalent resistance of the parallel resistances.
Substitute
Therefore, the equivalent resistance across any two vertices of the equilateral triangle is
So, the correct answer is “Option C”.
Note:
One can also draw the equivalent circuit diagram with resistance of one edge in the upper arm and of two edges (in series) in the lower arm and do the needful calculations to determine the equivalent resistance. Both methods will result with the same option.
Formula used:
The equivalent resistance of the two resistors connected in series is
Here,
The equivalent resistance of the two resistors connected in parallel is
Here,
Complete step by step answer:
The equilateral triangle is a triangle in which all of its three edges are of the same length.
The total resistance across any wire is directly proportional to the length of the wire.
The total resistance of the given wire is
The given wire is bent to form an equilateral triangle. Hence, the total resistance
Hence, the resistance through each edge of the equilateral triangle is
Now, calculate the resistance across any two vertices of the equilateral triangle.
The resistance across any two vertices of the equilateral triangle should be different as across any two vertices of the equilateral triangle two resistances are in series.
The diagram representing the resistances across 3 edges of the equilateral triangle and its equivalent circuit is as follows:

From the above diagram, it is clear that when a wire with resistance is bent into a equilateral triangle, its resistance divides equally in three edges of triangle and the its equivalent circuit across two vertices of the triangle contains resistance of the two edges of the triangle in series and resistance of one edge in parallel to them.
Let the two resistors in series be labeled
Calculate the equivalent resistance
Substitute
Hence, the equivalent resistance across the two parallel resistors is
Now, calculate the equivalent
Rewrite equation (2) for the equivalent resistance of the parallel resistances.
Substitute
Therefore, the equivalent resistance across any two vertices of the equilateral triangle is
So, the correct answer is “Option C”.
Note:
One can also draw the equivalent circuit diagram with resistance of one edge in the upper arm and of two edges (in series) in the lower arm and do the needful calculations to determine the equivalent resistance. Both methods will result with the same option.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE




