
A transparent solid cylindrical rod has a refractive index of
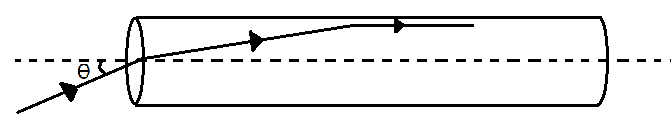
(A)
(B)
(C)
(D)

Answer
498k+ views
1 likes
Hint: The angle at which the ray is incident on the inside of the rod is the critical angle. As the sine of the critical angle is the inverse of the refractive index, we can get the critical angle. Now from geometry, we get the angle of refraction. So from Snell’s law, we can get the angle
Formula Used: In this solution we will be using the following formula,
where
Complete step by step solution:
To find the answer we first need to redraw the diagram as,
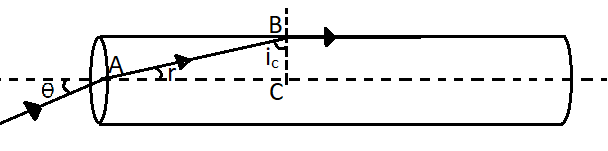
We can see that the ray inside the cylindrical rod strikes at point B at an angle such that the angle of refraction is
So substituting the values we get,
So we get, the critical angle as,
Since,
So we get,
Now from the triangle ABC, we can see that the sum of the critical angle and the angle of refraction is
Therefore,
Now substituting the values we get,
Now again we can apply the Snell’s law at the point A. Here, the first medium has a refractive index of
So using the Snell’s law we get,
So we get,
Since the value of
Substituting we get,
Therefore,
So we get the angle as,
So the correct answer is option (D).
Note:
The Snell’s law is a formula that is used to describe the angle of incidence to the angle of refraction. The ratio of the sine of the two angles can also be written as the ratio of the phase velocity of the wave in the two media.
Formula Used: In this solution we will be using the following formula,
where
Complete step by step solution:
To find the answer we first need to redraw the diagram as,

We can see that the ray inside the cylindrical rod strikes at point B at an angle such that the angle of refraction is
So substituting the values we get,
So we get, the critical angle as,
Since,
So we get,
Now from the triangle ABC, we can see that the sum of the critical angle and the angle of refraction is
Therefore,
Now substituting the values we get,
Now again we can apply the Snell’s law at the point A. Here, the first medium has a refractive index of
So using the Snell’s law we get,
So we get,
Since the value of
Substituting we get,
Therefore,
So we get the angle as,
So the correct answer is option (D).
Note:
The Snell’s law is a formula that is used to describe the angle of incidence to the angle of refraction. The ratio of the sine of the two angles can also be written as the ratio of the phase velocity of the wave in the two media.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE




