Question

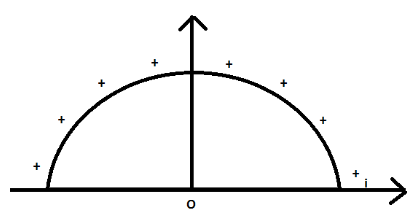
A thin semi-circular ring of radius R has a positive charge q distributed uniformly over it. The net field $$\overrightarrow{E}$$ at the centre O is:

$$A.{\displaystyle \frac{q}{4{\pi }^2{ϵ}_0{R}^2}}\hat{j}$$
$$B.{\displaystyle \frac{q}{4{\pi }^2{ϵ}_0{R}^2}}\hat{j}$$
$$C.{\displaystyle \frac{q}{2{\pi }^2{ϵ}_0{R}^2}}\hat{j}$$
$$D.{\displaystyle \frac{q}{2{\pi }^2{ϵ}_0{R}^2}}\hat{j}$$

Answer 1

Hint:Here, we first find out the linear charge density of the semicircular ring and then taking a charge element on the semi-circular ring, we find the electric field element for that charge element. Now, presuming that the whole semi circular ring is made up of such charge elements, we find out the total electric field by integrating the electric field elements.

Complete step by step answer:
Here, we take the radius of the semi-circular ring as R. Now, we suppose that $$\lambda$$ is the linear charge density of the semi-circular ring. The circumference of the ring is $$\pi R$$, so the linear charge density would be:
$$\lambda ={\displaystyle \frac{q}{\pi R}}$$
And also we know that. Now for a small charge dq on the wire with an angle of $$d\theta$$, then the charge dq in terms of linear charge density is given as:
$$dq=\lambda Rd\theta$$
Now, the electric field due to the charges which are uniformly distributed over the semicircular ring would be the integral of the electric field developed due to all the charges dq present and we will have to take the cosine component of these electric fields. Thus, the total electric field due to these charges is shown as below:
$$\overrightarrow{E}={\int }_{{\displaystyle \frac{-\pi }{2}}}^{{\displaystyle \frac{\pi }{2}}}dE\cos \theta$$
Here, dE can be obtained through the Coulomb’s law which is given as below:
$$E={\displaystyle \frac{kq}{r^2}}$$
Here, E is the electric field and q is the charge, while r is the distance between the charges. Thus,
$$\overrightarrow{E}={\int }_{{\displaystyle \frac{-\pi }{2}}}^{{\displaystyle \frac{\pi }{2}}}{\displaystyle \frac{k(\lambda Rd\theta )}{R^2}}\cos \theta (-\hat{j})$$
$$\Rightarrow \overrightarrow{E}=2\times {\displaystyle \frac{k\lambda }{R}}{\int }_{{\displaystyle \frac{-\pi }{2}}}^{{\displaystyle \frac{\pi }{2}}}\cos \theta d\theta (-\hat{j})$$
$$\Rightarrow \overrightarrow{E}=2\times {\displaystyle \frac{kq}{\pi R^2}}{\left[\sin \theta \right]}_0^{{\displaystyle \frac{\pi }{2}}}(-\hat{j})$$
$$\therefore \overrightarrow{E}={\displaystyle \frac{q}{2{\pi }^2{ϵ}_0{R}^2}}(-\hat{j})$$

Hence, option C is the correct answer.

Note: If instead of a semi circular ring, there was a full circular ring, then the electric field would have been half to that of a semi circular ring. Additionally, the electric field inside the circular ring would have been zero, and so as for the semicircular ring. This formula applies to the electric field outside the ring.