
A semi-infinite insulating rod has linear charge density

(A)
(B)
(C)
(D)

Answer
483.6k+ views
Hint: We will calculate the x-component and the y-component of the electric field at the point P, as shown in the figure, due to the semi-infinite insulating rod carrying uniform linear charge of density
Complete step by step answer:
It has been given that a semi-infinite insulating rod has linear charge density
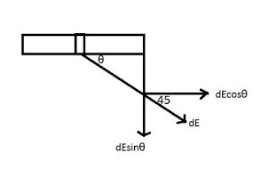
Consider an infinitesimal charge element of length
As the direction of electric field due to a charge element is along the line joining the element to the point where the field is to be calculated.
From the figure, the x-component of the field will be,
Integrating
For simplicity we take
Thus,
On simplifying the equation, we can get,
By rules of integration,
Substituting, the value of
We note that
Therefore, the angle that the electric field vector makes at P is
Since
It implies that
Now, the net electric field is given by,
Hence the net electric field is
The correct answer is Option C.
Note:
Electric field is defined as the electric force per unit charge. The direction of the field is taken to be the direction of the force it would exert on a positive test charge. The electric field is radially outward from a positive charge and radially in toward a negative point charge.
Complete step by step answer:
It has been given that a semi-infinite insulating rod has linear charge density

Consider an infinitesimal charge element of length
As the direction of electric field due to a charge element is along the line joining the element to the point where the field is to be calculated.
From the figure, the x-component of the field will be,
Integrating
For simplicity we take
Thus,
On simplifying the equation, we can get,
By rules of integration,
Substituting, the value of
We note that
Therefore, the angle that the electric field vector makes at P is
Since
It implies that
Now, the net electric field is given by,
Hence the net electric field is
The correct answer is Option C.
Note:
Electric field is defined as the electric force per unit charge. The direction of the field is taken to be the direction of the force it would exert on a positive test charge. The electric field is radially outward from a positive charge and radially in toward a negative point charge.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE




