
A point charge

(A) The electric flux passing through the curved surface of the hemisphere is
(B) The component of the electric field normal to the flat surface is constant over the surface
(C) Total flux through the curved and the flat surfaces is
(D) The circumference of the flat surface is an equipotential

Answer
501.6k+ views
Hint:To find electric flux we use Gauss’ Law. Electric flux is the measure of the electric field through a given area. The electric flux
Complete step by step solution:
Given: radius of the hemisphere is
The solid angle subtended by the flat surface at
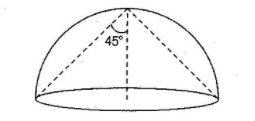
From the above figure, we can say that
Flux passing through curved surface is given by,
Put the value of equation
Hence, the option (A) is correct.
The component of electric field normal to the flat surface is
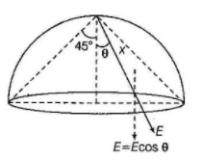
Here
The total flux due to charge
Potential at any point on the circumference of the flat surface is given by,
Since, the circumference is equidistant from charge
Therefore option (D) is correct.
Note:An equipotential surface is that at every point electrical potential is the same. The electric field
Complete step by step solution:
Given: radius of the hemisphere is
The solid angle subtended by the flat surface at

From the above figure, we can say that
Flux passing through curved surface is given by,
Put the value of equation
Hence, the option (A) is correct.
The component of electric field normal to the flat surface is

Here
The total flux due to charge
Potential at any point on the circumference of the flat surface is given by,
Since, the circumference is equidistant from charge
Therefore option (D) is correct.
Note:An equipotential surface is that at every point electrical potential is the same. The electric field
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE




