
(A) Obtain an expression for the mutual inductance between a long straight wire and a square loop of side a as shown in fig.
(B) Now assume that the straight wire carries a current of 50 A and the loop is moved to the right with a constant velocity,
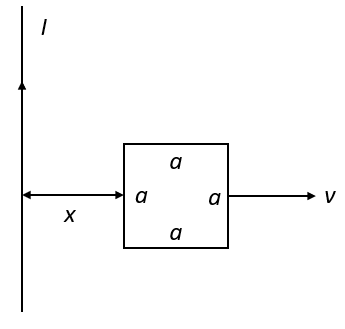

Answer
502.2k+ views
1 likes
Hint: Calculate the magnetic flux associated with the small vertical strip of the square loop and then integrate this magnetic flux from
Formula used:
Here, B is the magnetic field, I is the current, and r is the distance between the wire and square loop.
Here, L is the length of the side of the loop and v is the velocity of the loop.
Complete step by step answer:We know that the magnetic field due to current carrying wire at a distance r from the wire is,
Here, I is the current flowing through the long straight wire.
Now, consider the strip of small width
Here, dA is the area of the small strip dr.
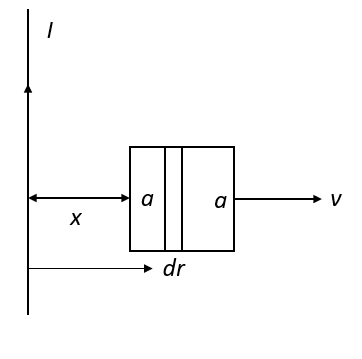
Substitute equation (1) in equation (2).
Integrate the above equation from x to
We know the relation between magnetic flux and mutual inductance,
Substitute the value of magnetic flux in the above equation.
This is the expression for the mutual inductance between the current carrying wire and square loop of side a.
(B)
The expression for induced emf in the loop due to current carrying wire is,
Here, L is the length of the side of the loop and v is the velocity of the loop.
The magnetic field due to current carrying wire at a distance x from the wire is,
Substitute the above equation in equation (3).
Substitute 50 A for I,
Therefore, the induced emf in the loop is
Note:To calculate the total flux associated with the square loop, integrate the magnetic field from x to
Formula used:
Here, B is the magnetic field, I is the current, and r is the distance between the wire and square loop.
Here, L is the length of the side of the loop and v is the velocity of the loop.
Complete step by step answer:We know that the magnetic field due to current carrying wire at a distance r from the wire is,
Here, I is the current flowing through the long straight wire.
Now, consider the strip of small width
Here, dA is the area of the small strip dr.

Substitute equation (1) in equation (2).
Integrate the above equation from x to
We know the relation between magnetic flux and mutual inductance,
Substitute the value of magnetic flux in the above equation.
This is the expression for the mutual inductance between the current carrying wire and square loop of side a.
(B)
The expression for induced emf in the loop due to current carrying wire is,
Here, L is the length of the side of the loop and v is the velocity of the loop.
The magnetic field due to current carrying wire at a distance x from the wire is,
Substitute the above equation in equation (3).
Substitute 50 A for I,
Therefore, the induced emf in the loop is
Note:To calculate the total flux associated with the square loop, integrate the magnetic field from x to
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




