
A man 1.8 m tall stands at a distance of 3.6 m from a lamp post and casts a shadow of 5.4m on the ground. Find the height of the lamp post.
Answer
530.4k+ views
Hint – In this question consider CD as the height of man and AB as the height of the tower (see figure). Use
Complete step-by-step answer:
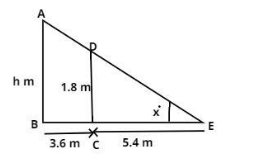
Let AB be the lamp post whose height be (h) meter as shown in figure.
Let DC be the man standing at 3.6 meter from the lamp post whose height is 1.8 meter as shown in figure.
The CE is the shadow of the man whose length is 5.4 meter as shown in figure.
So in triangle CDE, let
Now in triangle ABE we have,
Now substitute the values we have,
So the height of the lamp post is 3 meter.
So this is the required answer.
Hence option (C) is correct.
Note – Diagrammatic representation using the information provided in the question always helps in understanding the basic geometry of the problems. It is always advised to remember the direct formula for basic trigonometric ratios just like tan is the ratio of perpendicular and base. Key point here is that in such height and distance based problems the trigonometric ratio of
Complete step-by-step answer:

Let AB be the lamp post whose height be (h) meter as shown in figure.
Let DC be the man standing at 3.6 meter from the lamp post whose height is 1.8 meter as shown in figure.
The CE is the shadow of the man whose length is 5.4 meter as shown in figure.
So in triangle CDE, let
Now in triangle ABE we have,
Now substitute the values we have,
So the height of the lamp post is 3 meter.
So this is the required answer.
Hence option (C) is correct.
Note – Diagrammatic representation using the information provided in the question always helps in understanding the basic geometry of the problems. It is always advised to remember the direct formula for basic trigonometric ratios just like tan is the ratio of perpendicular and base. Key point here is that in such height and distance based problems the trigonometric ratio of
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
What is the Full Form of ISI and RAW

Which of the following districts of Rajasthan borders class 9 social science CBSE

Difference Between Plant Cell and Animal Cell

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

Discuss what these phrases mean to you A a yellow wood class 9 english CBSE




