
A field is in the shape of a pentagon as shown in the figure. Three of the interior angles of the pentagon are right angles. The remaining two interior angles are congruent. What is the measure of each angle?
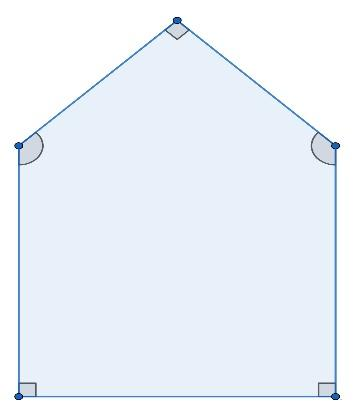

Answer
533.4k+ views
Hint: For solving this question firstly we will draw the given figure and consider there is a pentagon ABCDE as per the given configurations. After that, we will take a point O inside the pentagon and join every vertex of it to point O. Then, we will get five triangles and we will use one of the important result that the sum of the interior angles of a triangle is always
Given:
It is given that there is a field that is in the shape of a pentagon as shown in the figure. Three of the interior angles of the pentagon are right angles. The remaining two interior angles are congruent, and we have to find the measure of each angle.
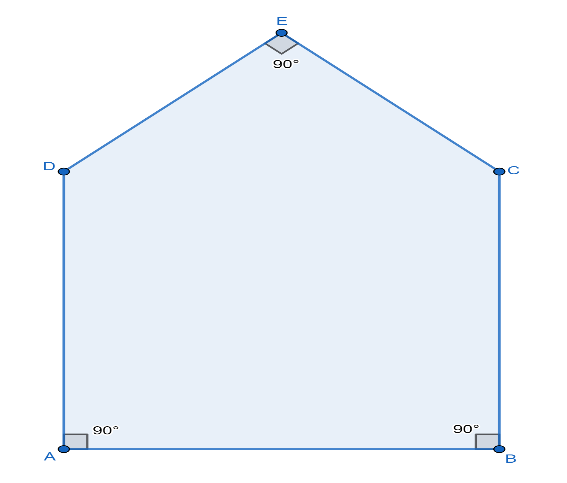
Now, let there be a pentagon ABCDE in which
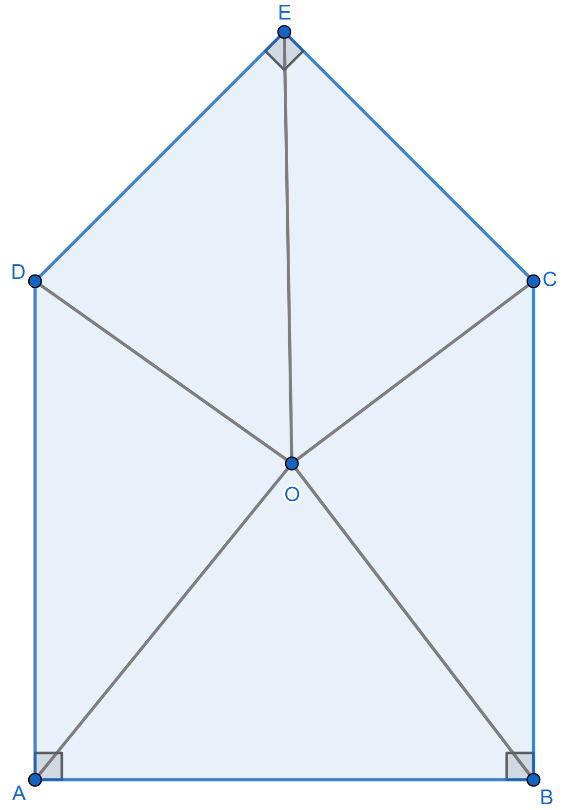
Now, just take a point O inside of the pentagon and join the point O with each vertex of the pentagon as shown in the figure below:
Now, as we know that sum of the interior angles of a triangle is
Now, as the lines are concurrent and intersect at point O. So, the value of the
Now, from the above result, we conclude that the value of
Thus, in the given pentagon there will be three angles of
Note: Here, we should first understand what is asked in the question and then proceed in the right direction to the correct answer quickly. Moreover, for objective problems, we should use the formula
Given:
It is given that there is a field that is in the shape of a pentagon as shown in the figure. Three of the interior angles of the pentagon are right angles. The remaining two interior angles are congruent, and we have to find the measure of each angle.
Now, let there be a pentagon ABCDE in which

Now, just take a point O inside of the pentagon and join the point O with each vertex of the pentagon as shown in the figure below:

Now, as we know that sum of the interior angles of a triangle is
Now, as the lines are concurrent and intersect at point O. So, the value of the
Now, from the above result, we conclude that the value of
Thus, in the given pentagon there will be three angles of
Note: Here, we should first understand what is asked in the question and then proceed in the right direction to the correct answer quickly. Moreover, for objective problems, we should use the formula
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
What is the Full Form of ISI and RAW

Difference Between Plant Cell and Animal Cell

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

What is 85 of 500 class 9 maths CBSE

Name 10 Living and Non living things class 9 biology CBSE




